Home /
Expert Answers /
Physics /
0-lt-theta-lt-theta-0-0-lt-phi-lt-2-pi-a-the-line-element-of-which-is-ds-2-a-2-d-th-pa728
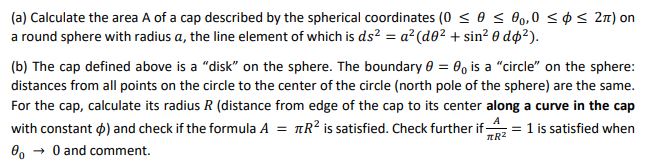
(Solved): 0<=\theta <=\theta _(0),0<=\phi <=2\pi a, the line element of which is ds^(2)=a^(2)(d\th ...
0<=\theta <=\theta _(0),0<=\phi <=2\pi a, the line element of which is ds^(2)=a^(2)(d\theta ^(2)+sin^(2)\theta d\phi ^(2)).
(b) The cap defined above is a "disk" on the sphere. The boundary \theta =\theta _(0) is a "circle" on the sphere:
distances from all points on the circle to the center of the circle (north pole of the sphere) are the same.
For the cap, calculate its radius R\phi A=\pi R^(2) is satisfied. Check further if (A)/(\pi R^(2))=1 is satisfied when
\theta _(0)->0 and comment.