(Solved): (15 points) The joint pdf of \( Y_{1} \) and \( Y_{2} \) is \[ f_{Y_{1}, Y_{2}}\left(y_{1}, y_{2}\r ...

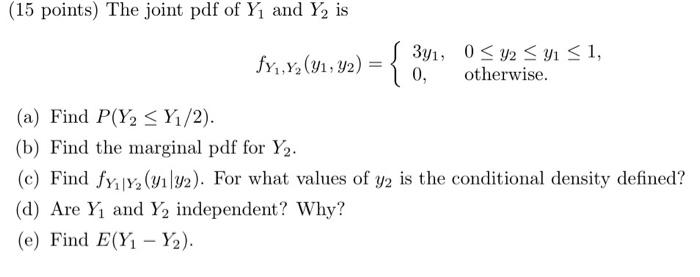
(15 points) The joint pdf of \( Y_{1} \) and \( Y_{2} \) is \[ f_{Y_{1}, Y_{2}}\left(y_{1}, y_{2}\right)=\left\{\begin{array}{ll} 3 y_{1}, & 0 \leq y_{2} \leq y_{1} \leq 1 \\ 0, & \text { otherwise } \end{array}\right. \] (a) Find \( P\left(Y_{2} \leq Y_{1} / 2\right) \) (b) Find the marginal pdf for \( Y_{2} \). (c) Find \( f_{Y_{1} \mid Y_{2}}\left(y_{1} \mid y_{2}\right) \). For what values of \( y_{2} \) is the conditional density defined? (d) Are \( Y_{1} \) and \( Y_{2} \) independent? Why? (e) Find \( E\left(Y_{1}-Y_{2}\right) \)