Home /
Expert Answers /
Economics /
15pts-consider-the-savings-function-sav-beta-0-beta-1-inc-u-u-sqrt-inc-e-where-e-is-a-ran-pa346
(Solved): (15pts) Consider the savings function sav=\beta _(0)+\beta _(1)inc+u,u=\sqrt(inc)*e where e is a ran ...
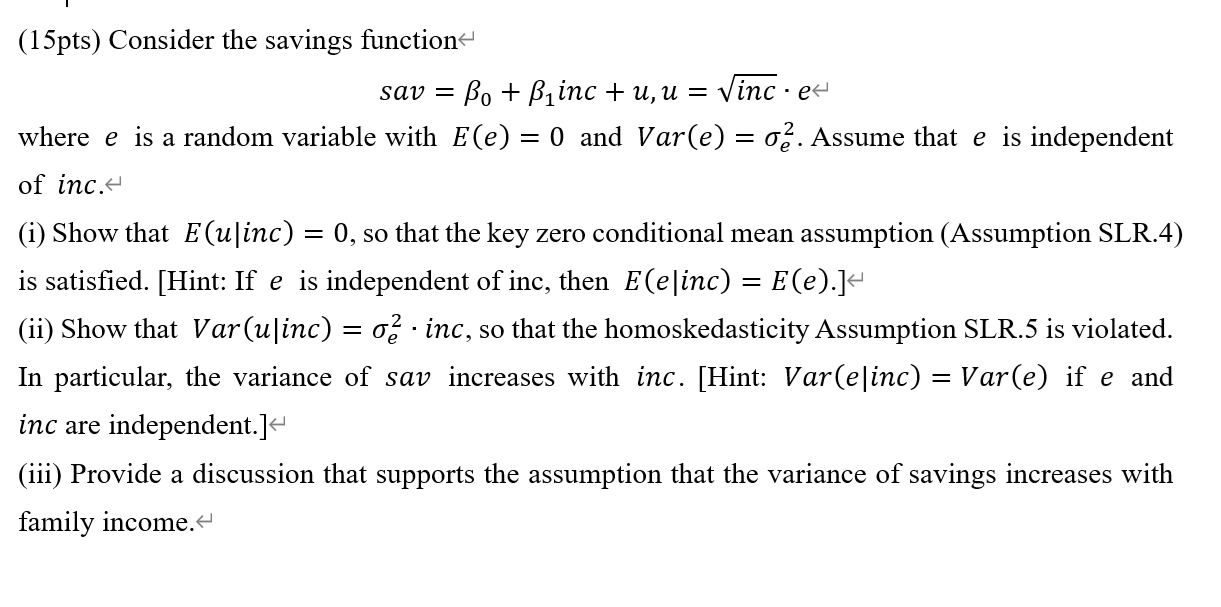
(15pts) Consider the savings function
sav=\beta _(0)+\beta _(1)inc+u,u=\sqrt(inc)*e
where e is a random variable with E(e)=0 and Var(e)=\sigma _(e)^(2). Assume that e is independent
of inc.
(i) Show that E(u|inc)=0e is independent of inc, then E(e|inc)=E(e).
(ii) Show that Var(u|inc)=\sigma _(e)^(2)*incVar(e|inc)=Var(e) if e and
inc are independent.