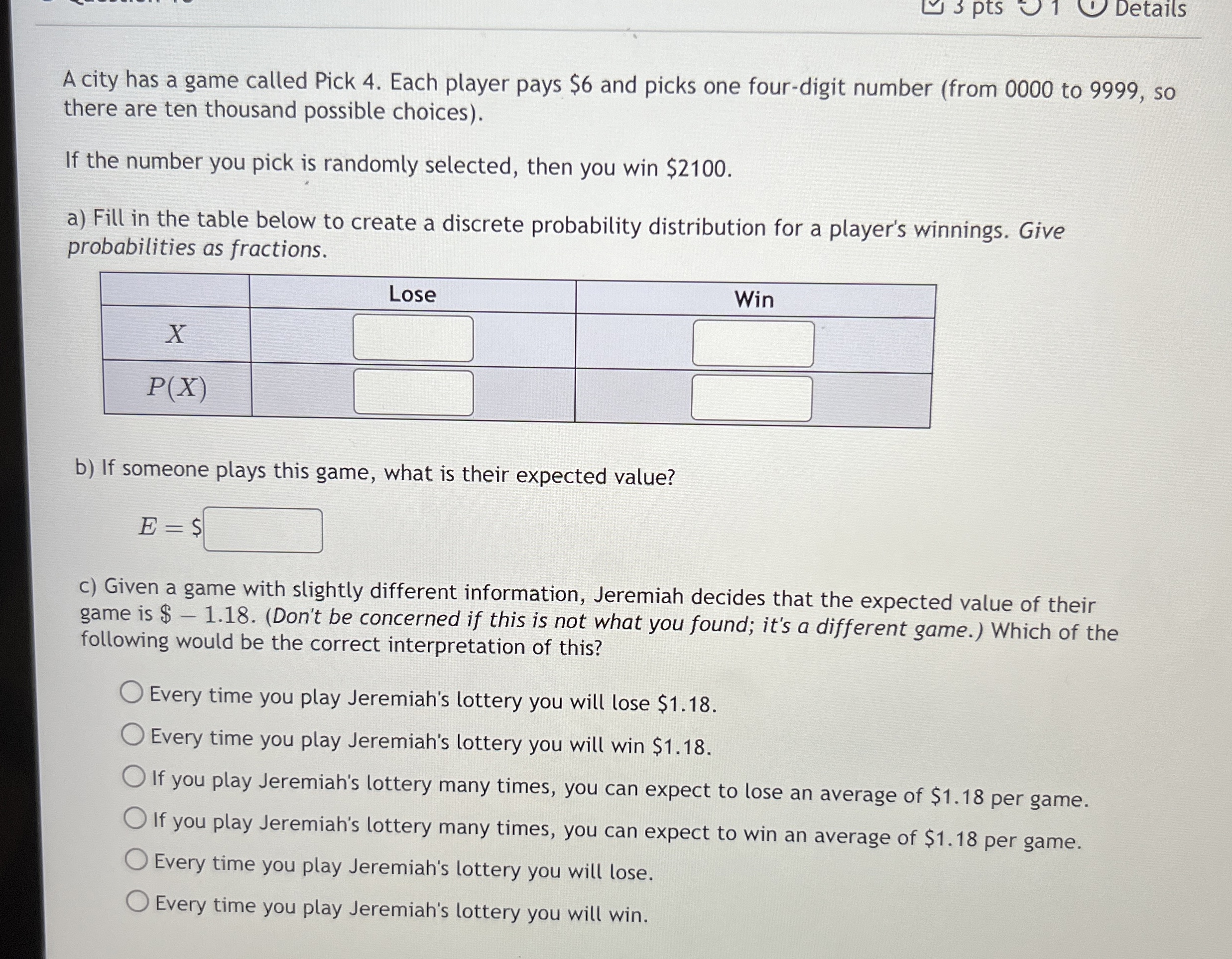
(Solved): 3 pts Details A city has a game called Pick 4. Each player pays $6 and picks one four-digit number ( ...

3 pts Details A city has a game called Pick 4. Each player pays

and picks one four-digit number (from 0000 to 9999 , so there are ten thousand possible choices). If the number you pick is randomly selected, then you win

. a) Fill in the table below to create a discrete probability distribution for a player's winnings. Give probabilities as fractions. \table[[,Lose,Win],[

,,],[

,,]] b) If someone plays this game, what is their expected value?

c) Given a game with slightly different information, Jeremiah decides that the expected value of their game is

. (Don't be concerned if this is not what you found; it's a different game.) Which of the following would be the correct interpretation of this? Every time you play Jeremiah's lottery you will lose

. Every time you play Jeremiah's lottery you will win

. If you play Jeremiah's lottery many times, you can expect to lose an average of

per game. If you play Jeremiah's lottery many times, you can expect to win an average of

per game. Every time you play Jeremiah's lottery you will lose. Every time you play Jeremiah's lottery you will win.