Home /
Expert Answers /
Electrical Engineering /
a-3-phi-100kva-460v-60hz-eight-pole-induction-machine-has-the-following-equivalent-circuit-paramet-pa745
(Solved): A 3\phi ,100kVA,460V,60Hz, eight-pole induction machine has the following equivalent circuit paramet ...
A 3\phi ,100kVA,460V,60Hz, eight-pole induction machine has the following equivalent circuit
parameters:
R_(1)=0.07\Omega ,x_(1)=0.2\Omega
R_(2)^(')=0.05\Omega ,x_(2)^(')=0.2\Omega
x_(m)=6.5\Omega ,
(a) Derive the Thevenin equivalent circuit for the induction machine.
(b) If the machine is connected to a 3\phi ,460V,60Hz supply, determine the starting torque, the
maximum torque the machine can develop, and the speed at which the maximum torque is
developed.
(c) If the maximum torque is to occur at start, determine the external resistance required in
each rotor phase. Assume a turns ratio (stator to rotor) of 1.2.
A 3\phi ,100kVA,460V,60Hz, eight-pole induction machine has the following equivalent circuit
parameters:
R_(1)=0.07\Omega ,x_(1)=0.2\Omega
R_(2)^(')=0.05\Omega ,
x_(m)=6.5\Omega
(a) Derive the Thevenin equivalent circuit for the induction machine.
(b) If the machine is connected to a 3\phi ,460V,60Hz supply, determine the starting torque, the
maximum torque the machine can develop, and the speed at which the maximum torque is
developed.
(c) If the maximum torque is to occur at start, determine the external resistance required in
each rotor phase. Assume a turns ratio (stator to rotor) of 1.2 .
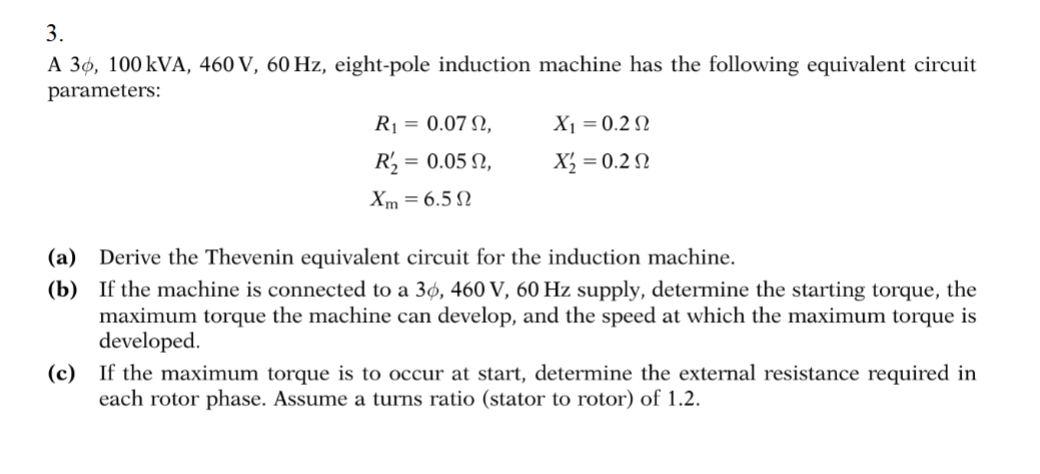
(a)
Per-phase voltage:
V_(1)=(V_(line ))/(\sqrt(3))
Thevenin equivalent circuit:
Voltage:
V_(th)=V_(1)\times (x_(m))/(x_(1)+x_(m))
Thevenin impedance:
Z_(th)=(jx_(m)\times (R_(1)+jx_(1)))/(R_(1)+j(x_(1)+x_(m)))
R_(th)
x_(th)
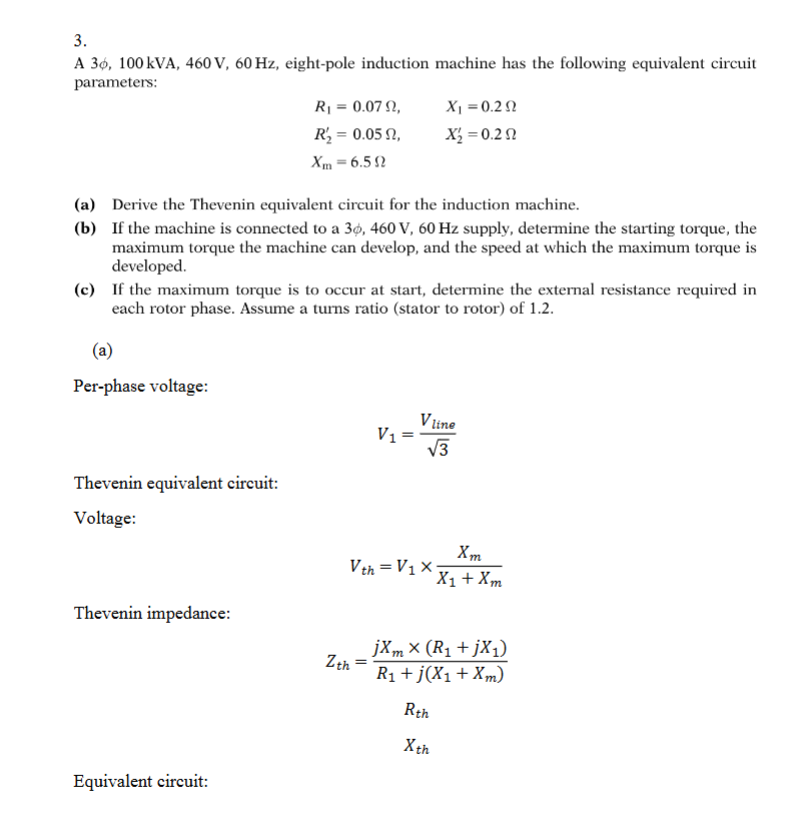
Equivalent circuit: (b)
Synchronous speed:
n_(s)=(120f)/(p)
Convert to rad per sec:
\omega =(2\pi n)/(60)s=1 I_(2)=(V_(th))/((R_(th)+(R^(')2)/(s))+jx_(th)+jx_(2)^('))
Air gap power:
P_(ag)=3(x)/(x)I _(()2)^(2)\times (R_(2)^('))/(s)
Starting torque:
T_(st)=(P_(ag))/(\omega )
Slip at maximum torque:
s_(Tmax)=(R_(2)^('))/(\sqrt(R_(th)^(2)+(x_(th)+x_(2)^('))^(2)))
Max torque:
T_(max)=(3)/(2\omega )\times (V_(th)^(2))/(\sqrt(R_(th)^(2)+(x_(th)+x^('))^(2))+R_(th))
Speed:
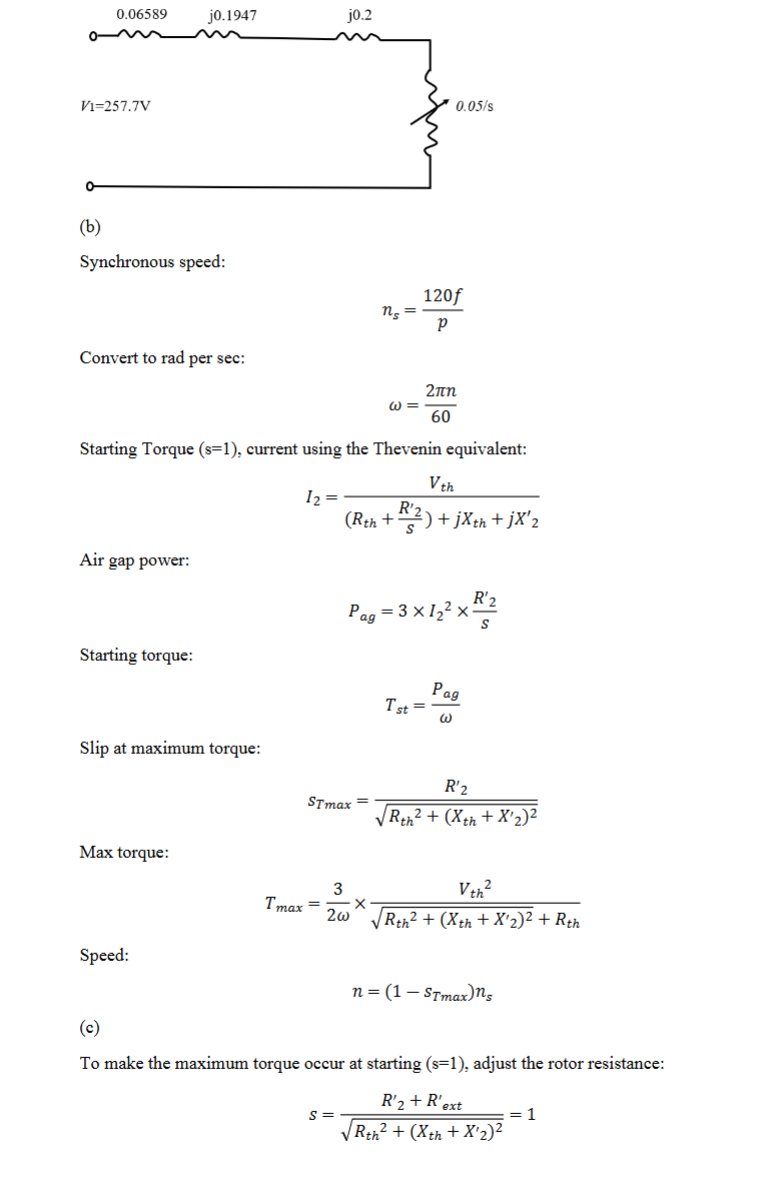
n=(1-s_(Tmax))n_(s)s=1 s=(R_(2)^(')+R_(ext)^('))/(\sqrt(R_(th)^(2)+(x_(th)+x_(2)^('))^(2)))=1 Therefore:
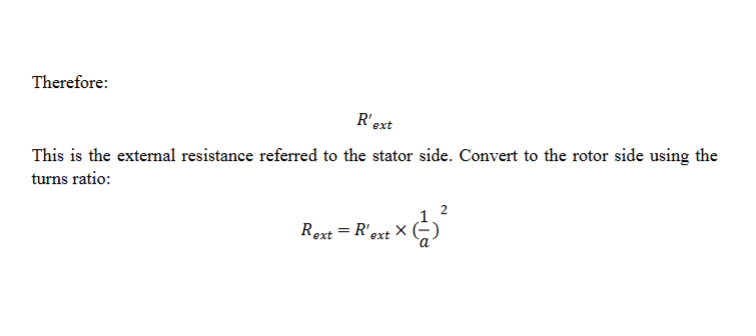
R_(ext )^(')
This is the external resistance referred to the stator side. Convert to the rotor side using the
turns ratio:
R_(ext)=R_(ext)^(')\times ((1)/(a))^(2)