(Solved): (b) Let A and D be nonsingular matrices of orders k and m, respectively, B be k\times m, and C be k\ ...

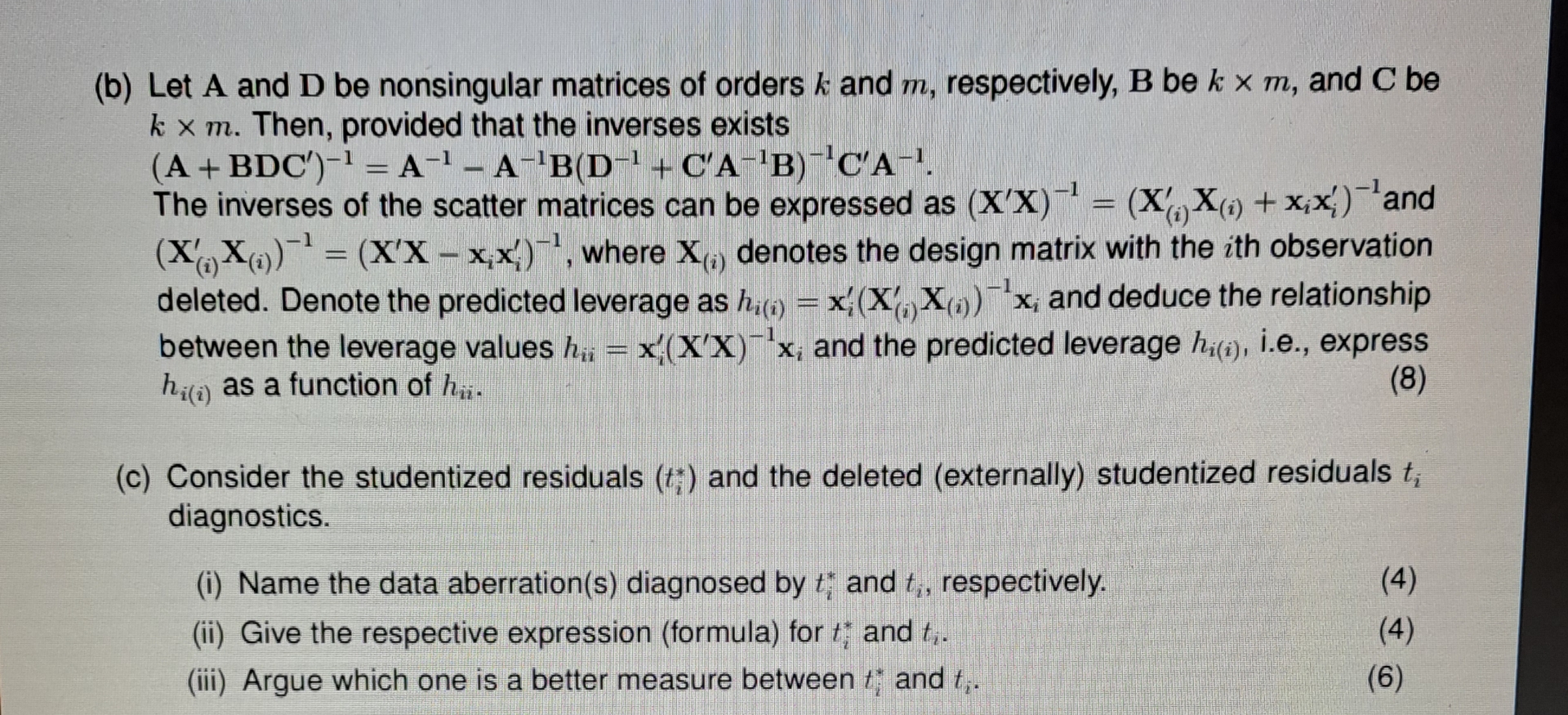
(b) Let

and

be nonsingular matrices of orders

and

, respectively,

be

, and

be

. Then, provided that the inverses exists

. The inverses of the scatter matrices can be expressed as

and

, where

denotes the design matrix with the

th observation deleted. Denote the predicted leverage as

and deduce the relationship between the leverage values

and the predicted leverage

, i.e., express

as a function of

. (c) Consider the studentized residuals (

) and the deleted (externally) studentized residuals

diagnostics. (i) Name the data aberration(s) diagnosed by

and

, respectively. (ii) Give the respective expression (formula) for

and

. (iii) Argue which one is a better measure between

and

. (6)