Home /
Expert Answers /
Calculus /
can-yiu-do-questions-2-and-3-nbsp-problem-2-10-points-given-y-t-x-left-begin-array-cc-x-pa997
(Solved): can yiu do questions 2 and 3 Problem(2) (10 points) Given \( y=T(x)=\left\{\begin{array}{cc}x^ ...
can yiu do questions 2 and 3
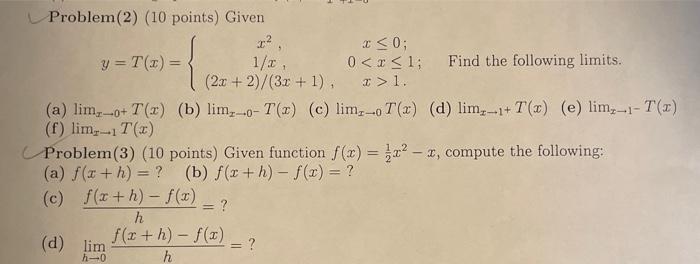
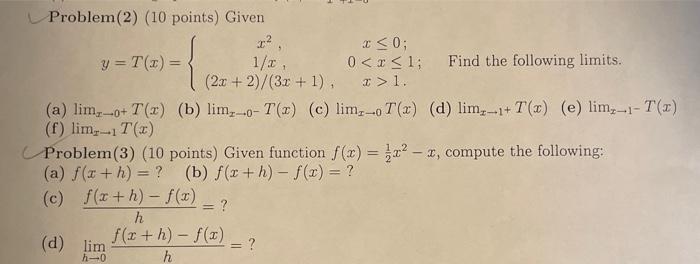
Problem(2) (10 points) Given \( y=T(x)=\left\{\begin{array}{cc}x^{2}, & x \leq 0 \\ 1 / x, & 01\end{array} \quad\right. \) Find the following limits. (a) \( \lim _{x \rightarrow 0^{+}} T(x) \) (b) \( \lim _{x \rightarrow 0^{-}} T(x) \) (c) \( \lim _{x \rightarrow 0^{2}} T(x) \) (d) \( \lim _{x \rightarrow 1^{+}} T(x) \) (e) \( \lim _{x \rightarrow 1^{-}} T(x) \) (f) \( \lim _{x \rightarrow 1} T(x) \) Problem(3) (10 points) Given function \( f(x)=\frac{1}{2} x^{2}-x \), compute the following: (a) \( f(x+h)= \) ? (b) \( f(x+h)-f(x)= \) ? (c) \( \frac{f(x+h)-f(x)}{h}= \) ? (d) \( \lim _{h \rightarrow 0} \frac{f(x+h)-f(x)}{h}= \) ?