(Solved): Consider An (to be the subgroup of Upper triangular matrices whose diagonal entries can be any n ...

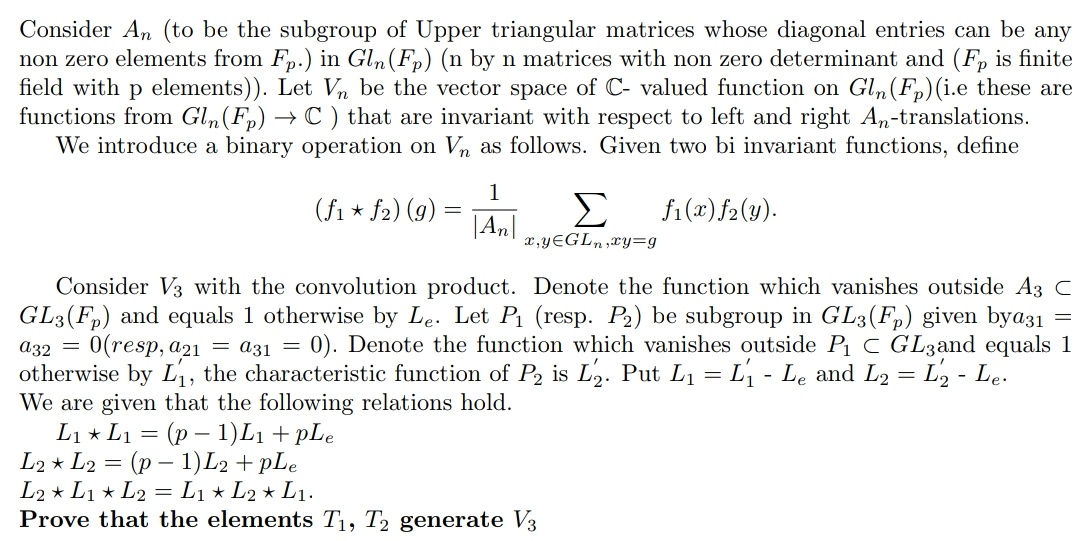
Consider $A_n$ (to be the subgroup of Upper triangular matrices whose diagonal entries can be any non zero elements from $F_p$.) in $G{l}_n\left(F_p\right)$ (n by n matrices with non zero determinant and $(F_p$ is finite field with p elements)). Let $V_n$ be the vector space of $\mathbb{C}$ - valued function on $G{l}_n\left(F_p\right)$ (i.e these are functions from $G{l}_n\left(F_p\right)\to \mathbb{C}$ ) that are invariant with respect to left and right $A_n$-translations. We introduce a binary operation on $V_n$ as follows. Given two bi invariant functions, define $\left(f_1\star f_2\right)(g)=\frac{1}{|A_n|}{\sum }_{x,y\in G{L}_n,xy=g}{f}_1(x)f_2(y).$ Consider $V_3$ with the convolution product. Denote the function which vanishes outside $A_3\subset$ $G{L}_3\left(F_p\right)$ and equals 1 otherwise by $L_e$. Let $P_1$ (resp. $P_2$ ) be subgroup in $G{L}_3\left(F_p\right)$ given by $a_{31}=$ $a_{32}=0($ resp,$a_{21}=a_{31}=0)$. Denote the function which vanishes outside $P_1\subset G{L}_3$ and equals 1 otherwise by $L_1^{\prime }$, the characteristic function of $P_2$ is $L_2^{\prime }$. Put $L_1=L_1^{\prime }-L_e$ and $L_2=L_2^{\prime }-L_e$. We are given that the following relations hold. $\begin{array}{c}{L}_1\star L_1=(p-1)L_1+p{L}_e\\ L_2\star L_2=(p-1)L_2+p{L}_e\\ L_2\star L_1\star L_2=L_1\star L_2\star L_1\end{array}$ Prove that the elements $T_1,T_2$ generate $V_3$