(Solved): Evaluate the function at the indicated values. (If an answer is undefined, enter UNDEFINED.) \[ \be ...

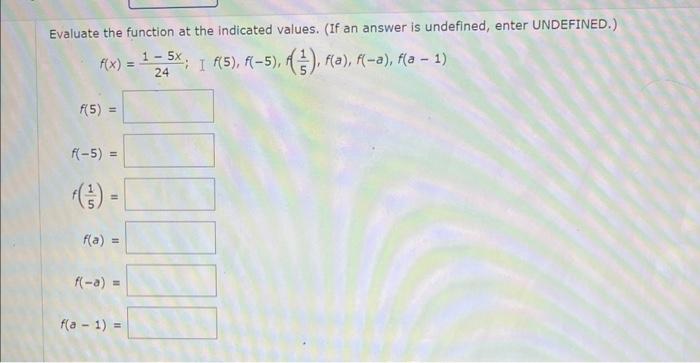
Evaluate the function at the indicated values. (If an answer is undefined, enter UNDEFINED.) \[ \begin{aligned} f(x) &=\frac{1-5 x}{24} ; I f(5), f(-5), f\left(\frac{1}{5}\right), f(a), f(-a), f(a-1) \\ f(5) &=\\ f(-5) &=\\ f\left(\frac{1}{5}\right) &=\\ f(a) &=\\ f(-a) &= \end{aligned} \] Evaluate the piecewise defined function at the indicated values. \[ f(x)=\left\{\begin{array}{ll} 8 x & \text { if } x<-2 \\ x+5 & \text { if }-2 \leq x \leq 2 \\ (x-2)^{2} & \text { if } x>2 \end{array}\right. \] Find the net change in the value of the function between the given inputs. \[ f(x)=4-8 x ; \text { from } 6 \text { to } 8 \] Find \( f(a), f(a+h) \), and the difference quotient \( \frac{f(a+h)-f(a)}{h} \), where \( h \neq 0 \) \[ f(x)=\frac{5 x}{x-4} \] \[ f(a)= \] \[ f(a+h)= \] \[ \frac{f(a+h)-f(a)}{h}= \] Find the domain of the function. (Enter your answer using interval notation.) \[ f(x)=\frac{x+7}{x^{2}-1} \] Find the domain of the function. (Enter your answer using interval notation.) \[ f(x)=\sqrt{4-9 x} \] Sketch the graph of the function by first making a table of values. (If an answer is undefined, enter UNDEFINED.) \[ g(x)=-(x+3)^{2} \] Sketch the graph. A graph of a piecewise defined function is given. Find a formula for the function in the indicated form. \[ f(x)=\left\{\begin{array}{ll} & \text { if } x \leq-1 \\ & \text { if }-12 \end{array}\right. \] Use the Vertical Line Test to determine whether the curve is the graph of a function of \( x \). Yes, the curve is a function of \( x \). No, the curve is not a function of \( x \). . If the curve is a function, state the domain and range. domain: \( [-4,1] \) \( \{-3\} \cup[0,3] \) \( \{-3\} \cup(0,3] \) \( (-\infty, \infty) \) The curve is not a function. 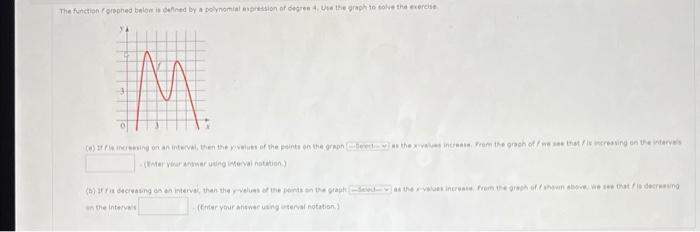wh the intorves (finter your aniswae ushy veqenal notation,) A graphing calculator is recommended. Solve the given equation or inequality graphically. State your answers rounded to two decimals. 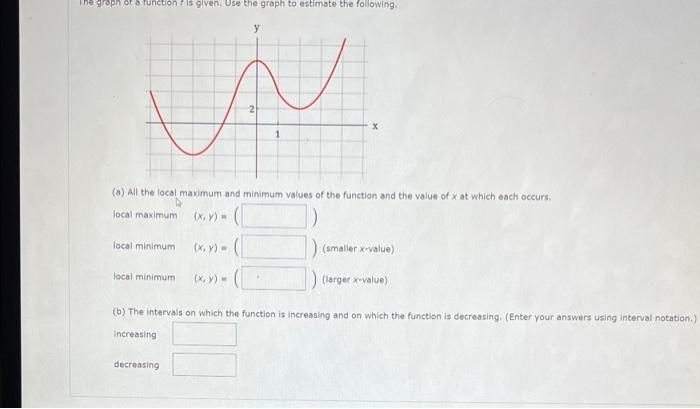(a) \( 5 x^{2}-x^{3}=-x^{2}+3 x+4 \) (Enter your answers as a comma-separated list.) \( x= \) (b) \( 5 x^{2}-x^{3} \leq-x^{2}+3 x+4 \) (Enter your answer using interval notation.) \( x= \) local minimum local minimum