(Solved): Evaluate the integral \( \int_{-\pi / 4}(\sin x+\cos x) d x \) using the fundamental theorem of cal ...

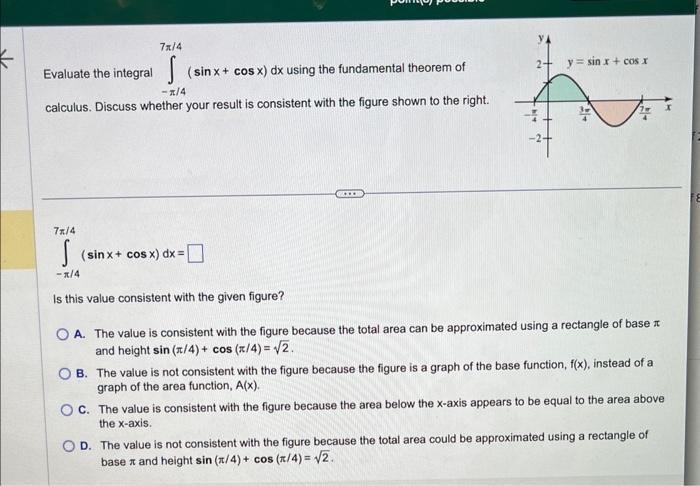
Evaluate the integral \( \int_{-\pi / 4}(\sin x+\cos x) d x \) using the fundamental theorem of calculus. Discuss whether your result is consistent with the figure shown to the right. \[ \int_{-\pi / 4}^{7 \pi / 4}(\sin x+\cos x) d x= \] Is this value consistent with the given figure? A. The value is consistent with the figure because the total area can be approximated using a rectangle of base \( \pi \) and height \( \sin (\pi / 4)+\cos (\pi / 4)=\sqrt{2} \). B. The value is not consistent with the figure because the figure is a graph of the base function, \( f(x) \), instead of a graph of the area function, \( A(x) \). C. The value is consistent with the figure because the area below the \( x \)-axis appears to be equal to the area above the \( x \)-axis. D. The value is not consistent with the figure because the total area could be approximated using a rectangle of base \( \pi \) and height \( \sin (\pi / 4)+\cos (\pi / 4)=\sqrt{2} \).