(Solved): explain the following concept Finding the Solid Boundary of the Cylinder (Assuming, Psi = 0) Now w ...
explain the following concept Finding the Solid Boundary of the Cylinder (Assuming, Psi = 0) Now we follow the essential steps involving the superposition of elementary flows in order to form a flow about the body of interest. A streamline has to be determined which encloses an area whose shape is of practical importance in fluid flow. This streamline will describe the boundary of a two-dimensional solid body. The remaining streamlines outside this solid region will constitute the flow about this body. Let us look for the streamline whose value is zero. Thus, we obtain
U_(0)y-(\chi sin\theta )/(r)=0replacing
yby
rsin\theta , we have
sin\theta (U_(0)r-(\chi )/(r))=0If
\theta =0or
\theta =\pi , the equation is satisfied. This indicates that the
xaxis is a part of the streamline
\psi =0. When the quantity in the parentheses is zero, the equation is identically satisfied. Hence it follows that
r=((\chi )/(U_(0)))^((1)/(2))Finding the Solid Boundary of the Cylinder (Assuming, Psi = 0) It can be said that there is a circle of radius
((\chi )/(U_(0)))^((1)/(2))which is an intrinsic part of the streamline
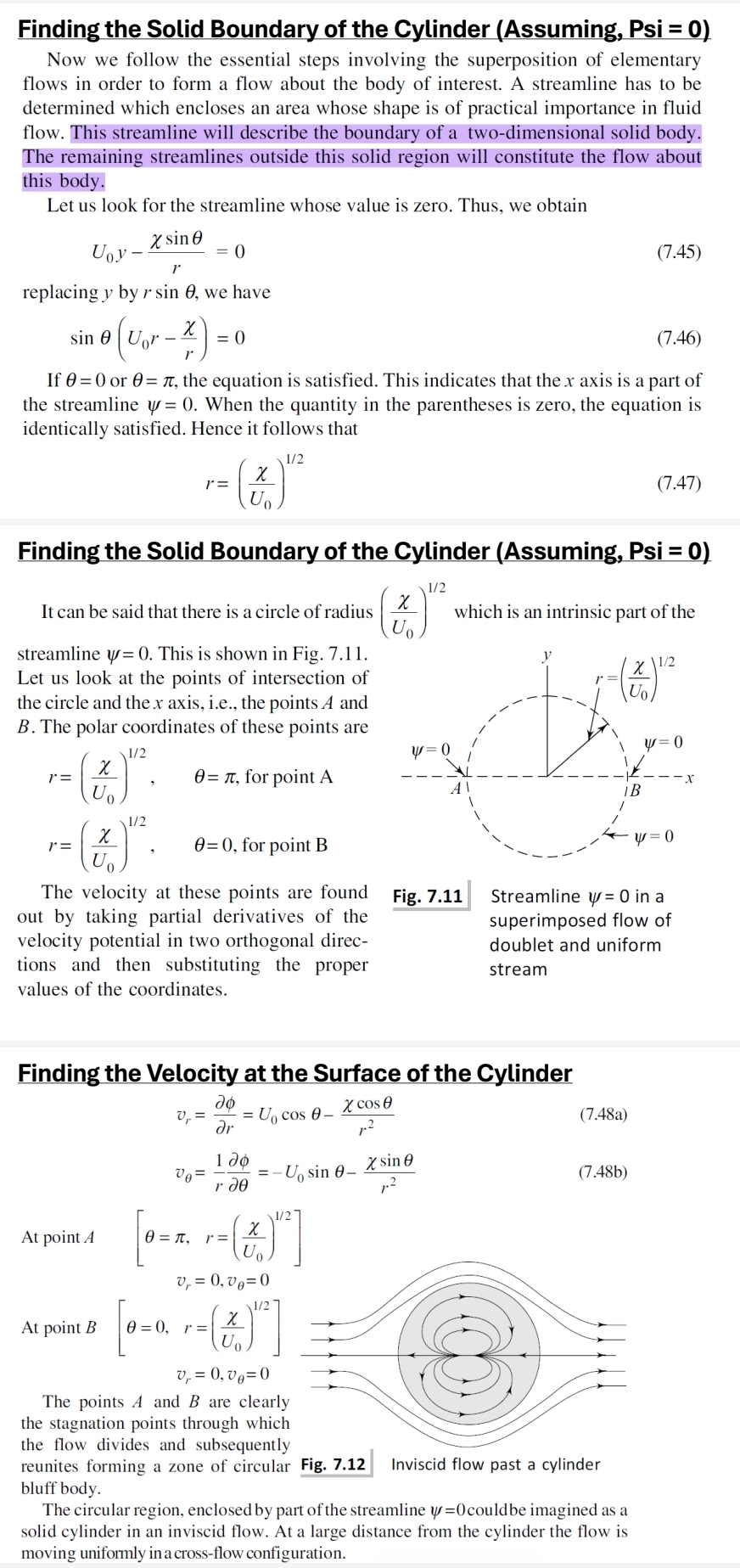
\psi =0. This is shown in Fig. 7.11. Let us look at the points of intersection of the circle and the
xaxis, i.e., the points
Aand
B. The polar coordinates of these points are
r=((\chi )/(U_(0)))^((1)/(2)),\theta =\pi , for point A
r=((\chi )/(U_(0)))^((1)/(2)),\theta =0, for point B The velocity at these points are found out by taking partial derivatives of the velocity potential in two orthogonal directions and then substituting the proper Fig. 7.11 Streamline
\psi =0in a superimposed flow of doublet and uniform stream Finding the Velocity at the Surface of the Cylinder
v_(r)=(del\phi )/(delr)=U_(0)cos\theta -(\chi cos\theta )/(r^(2))
v_(\theta )=(1)/(r)(del\phi )/(del\theta )=-U_(0)sin\theta -(\chi sin\theta )/(r^(2))At point