(Solved): Find p_(1) and p_(2), the prices per unit (in dollars), so as to maximize the total revenue R=x_(1)p ...
Find
p_(1)and
p_(2), the prices per unit (in dollars), so as to maximize the total revenue
R=x_(1)p_(1)+x_(2)p_(2), where
x_(1)and
x_(2)are the numbers of units sold, for a retail outlet that sells two substitute products with the given demand functions.
x_(1)=660-4p_(1)+2p_(2),x_(2)=870+Find
p_(1)and
p_(2), the prices per unit (in dollars), so as to maximize the total revenue
R=x_(1)p_(1)+x_(2)p_(2), where
x_(1)and
x_(2)are the numbers of units sold, for a retail outlet that sells two substitute products with the given demand functions.
,x_(1)=660-4p_(1)+2p_(2),x_(2)=870+4p_(1)-3p_(2)
p_(1)=$
p_(2)=$Need Help?
1-3p_(2)
p_(1)=$
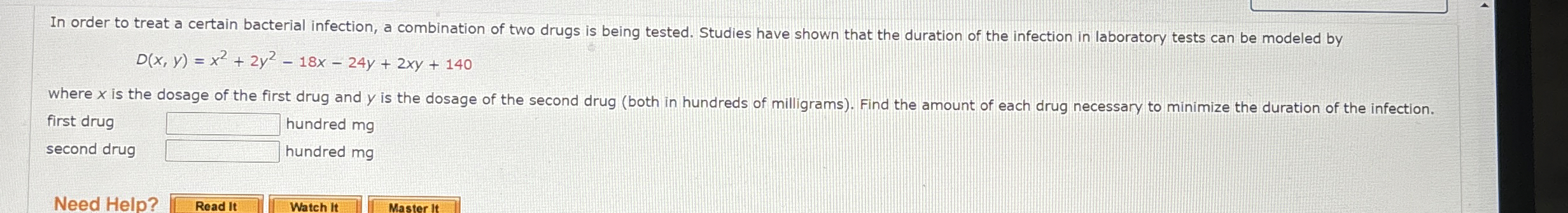
p_(2)=$Need Help? In order to treat a certain bacterial infection, a combination of two drugs is being tested. Studies have shown that the duration of the infection in laboratory tests can be modeled by
D(x,y)=x^(2)+2y^(2)-18x-24y+2xy+140where
xis the dosage of the first drug and
yis the dosage of the second drug (both in hundreds of milligrams). Find the amount of each drug necessary to minimize the duration of the infection. first drug hundred mg second drug hundred mg