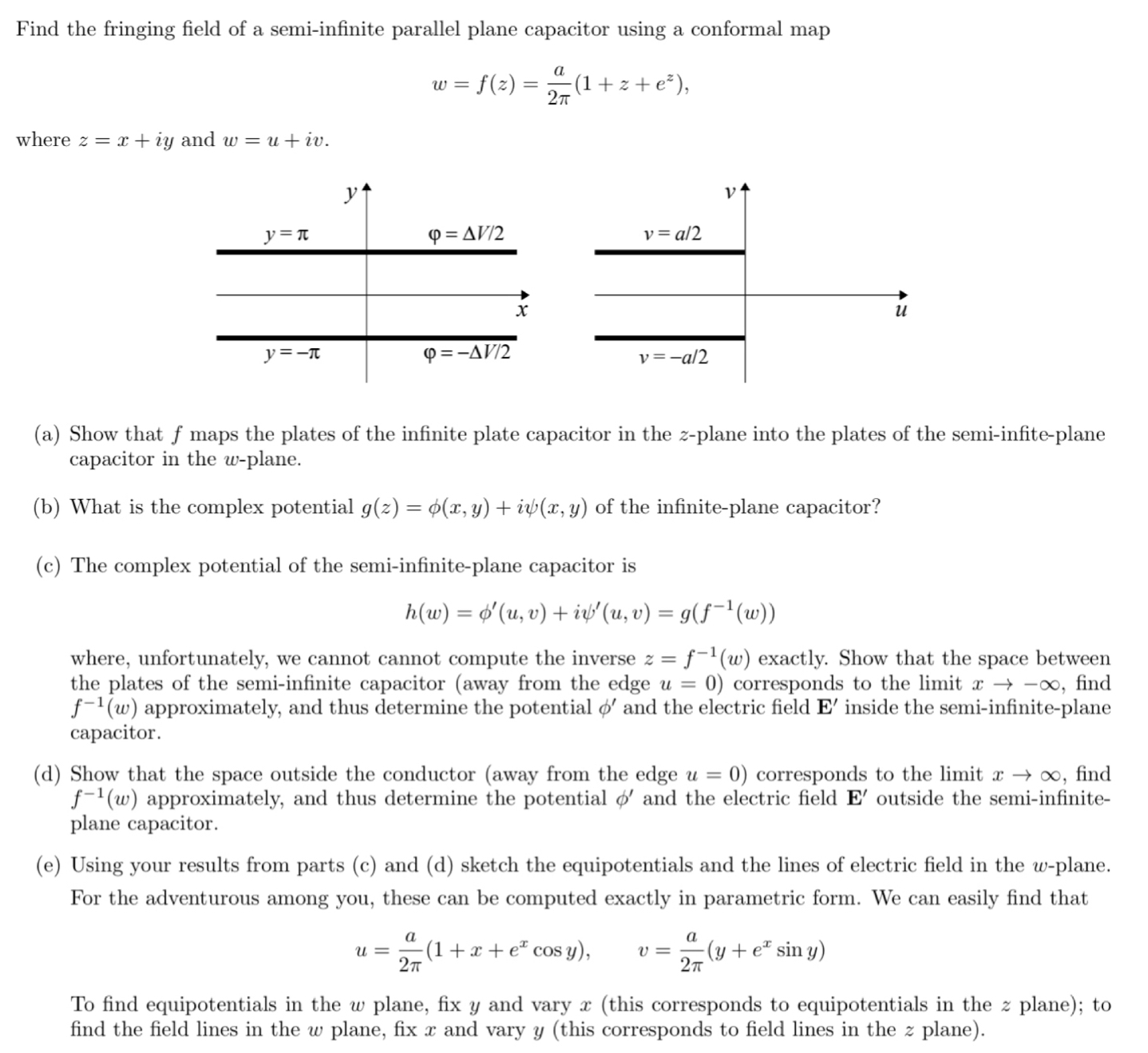
(Solved): Find the fringing field of a semi-infinite parallel plane capacitor using a conformal map w=f(z)=(a) ...
Find the fringing field of a semi-infinite parallel plane capacitor using a conformal map
w=f(z)=(a)/(2\pi )(1+z+e^(z))where
z=x+iyand
w=u+iv. (a) Show that
fmaps the plates of the infinite plate capacitor in the
z-plane into the plates of the semi-infite-plane capacitor in the
w-plane. (b) What is the complex potential
g(z)=\phi (x,y)+i\psi (x,y)of the infinite-plane capacitor? (c) The complex potential of the semi-infinite-plane capacitor is
h(w)=\phi ^(')(u,v)+i\psi ^(')(u,v)=g(f^(-1)(w))where, unfortunately, we cannot cannot compute the inverse
z=f^(-1)(w)exactly. Show that the space between the plates of the semi-infinite capacitor (away from the edge
u=0) corresponds to the limit
x->-\infty , find
f^(-1)(w)approximately, and thus determine the potential
\phi ^(')and the electric field
E^(')inside the semi-infinite-plane capacitor. (d) Show that the space outside the conductor (away from the edge
u=0) corresponds to the limit
x->\infty , find
f^(-1)(w)approximately, and thus determine the potential
\phi ^(')and the electric field
E^(')outside the semi-infiniteplane capacitor. (e) Using your results from parts (c) and (d) sketch the equipotentials and the lines of electric field in the
w-plane. For the adventurous among you, these can be computed exactly in parametric form. We can easily find that
u=(a)/(2\pi )(1+x+e^(x)cosy),v=(a)/(2\pi )(y+e^(x)siny)To find equipotentials in the
wplane, fix
yand vary
x(this corresponds to equipotentials in the
zplane); to find the field lines in the
wplane, fix
xand vary
y(this corresponds to field lines in the
zplane).