(Solved): Find the z value such that 95% of the standard normal curve lies between -z and z. Sketch the area ...

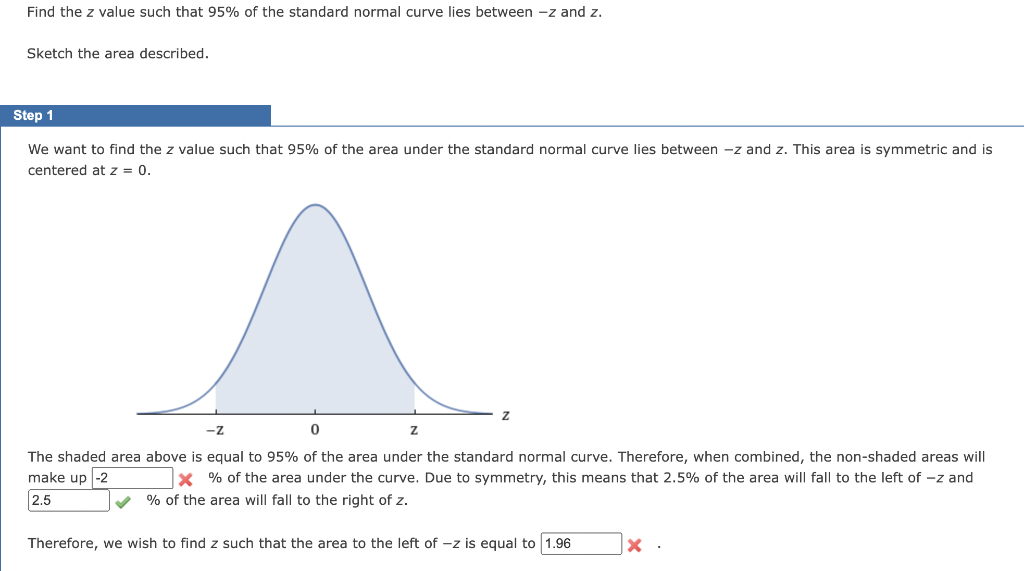
Find the z value such that 95% of the standard normal curve lies between -z and z. Sketch the area described. Step 1 We want to find the z value such that 95% of the area under the standard normal curve lies between -z and z. This area is symmetric and is centered at z = 0. A The shaded area above is equal to 95% of the area under the standard normal curve. Therefore, when combined, the non-shaded areas will make up -2 X% of the area under the curve. Due to symmetry, this means that 2.5% of the area will fall to the left of -z and % of the area will fall to the right of z. 2.5 -Z Z Therefore, we wish to find z such that the area to the left of -z is equal to 1.96 X