(Solved): For the following functions, determine the set of all points in which they are continuous. \[ f: \m ...

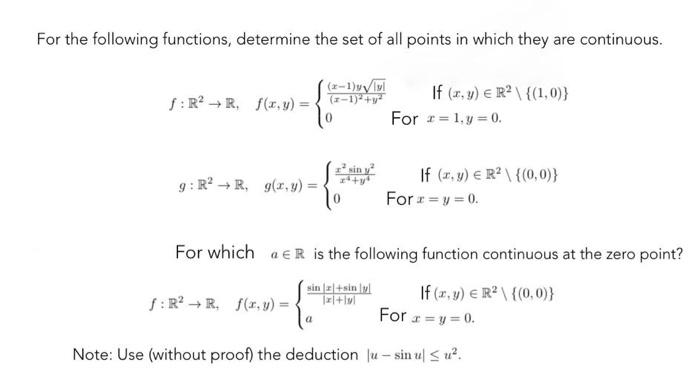
For the following functions, determine the set of all points in which they are continuous. \[ f: \mathbb{R}^{2} \rightarrow \mathbb{R}, \quad f(x, y)=\left\{\begin{array}{ll} \frac{(x-1) y \sqrt{|y|}}{(x-1)^{2}+y^{2}} & \text { If }(x, y) \in \mathbb{R}^{2} \backslash\{(1,0)\} \\ 0 & \text { For } x=1, y=0 . \end{array}\right. \] \( g: \mathbb{R}^{2} \rightarrow \mathbb{R}, \quad g(x, y)=\left\{\begin{array}{lc}\frac{x^{2} \sin y^{2}}{x^{4}+y^{4}} & \text { If }(x, y) \in \mathbb{R}^{2} \backslash\{(0,0)\} \\ 0 & \text { For } x=y=0 .\end{array}\right. \) For which \( a \in \mathbb{R} \) is the following function continuous at the zero point? \[ f: \mathbb{R}^{2} \rightarrow \mathbb{R}, \quad f(x, y)=\left\{\begin{array}{ll} \frac{\sin |x|+\sin |y|}{|x|+|y|} & \text { |f }(x, y) \in \mathbb{R}^{2} \backslash\{(0,0)\} \\ a & \text { For } x=y=0 . \end{array}\right. \] Note: Use (without proof) the deduction \( |u-\sin u| \leq u^{2} \).