(Solved): Formulate a linear programming model to cover the demand requirements with the minimum number of o ...

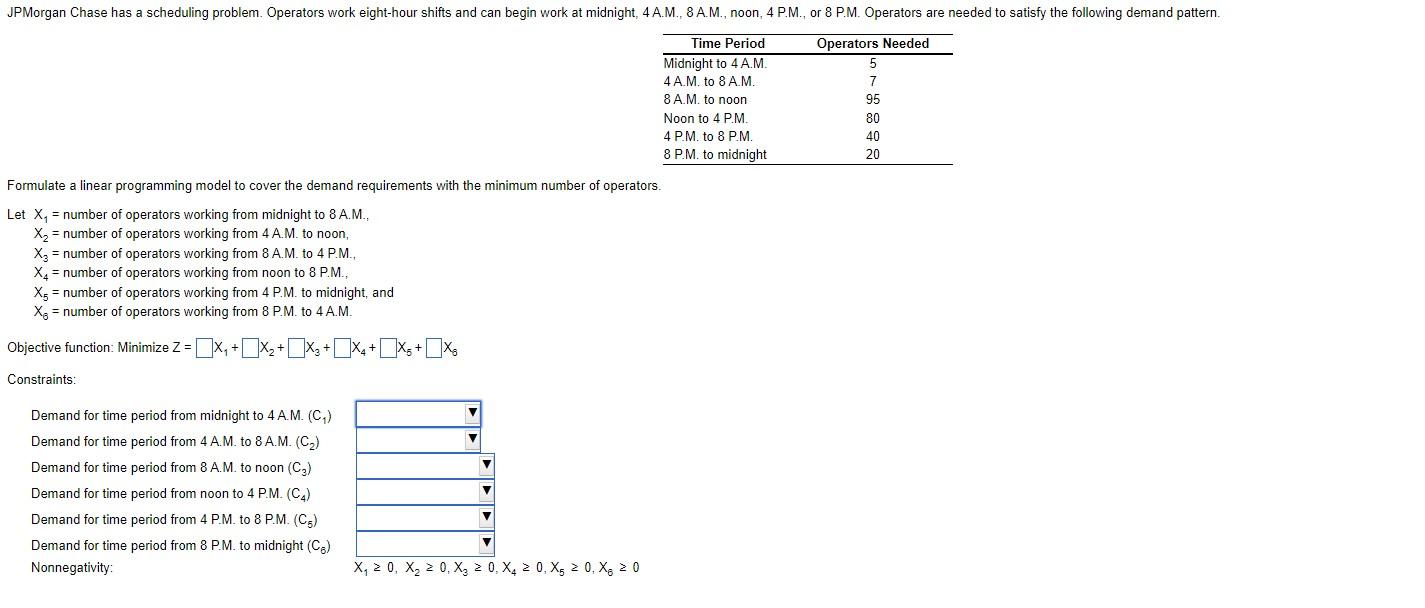
Formulate a linear programming model to cover the demand requirements with the minimum number of operators. Let \( \mathrm{X}_{1}= \) number of operators working from midnight to 8 A.M., \( X_{2}= \) number of operators working from 4 A.M. to noon, \( X_{3}= \) number of operators working from 8 A.M. to 4 P.M., \( \mathrm{X}_{4}= \) number of operators working from noon to 8 P.M., \( X_{5}= \) number of operators working from 4 P.M. to midnight, and \( X_{8}= \) number of operators working from 8 P.M. to 4 A.M. Objective function: Minimize \( Z=\quad x_{1}+{ }_{2}{ }^{+} \zeta_{3}+x_{4}+{ }_{5}+\square x_{8} \) Constraints: Demand for time period from midnight to 4 A.M. (C \( \left.\mathrm{C}_{1}\right) \) Demand for time period from 4 A.M. to 8 A.M. \( \left(\mathrm{C}_{2}\right) \) Demand for time period from 8 A.M. to noon \( \left(\mathrm{C}_{3}\right) \) Demand for time period from noon to 4 P.M. \( \left(\mathrm{C}_{4}\right) \) Demand for time period from 4 P.M. to 8 P.M. \( \left(\mathrm{C}_{5}\right) \) Demand for time period from 8 P.M. to midnight \( \left(\mathrm{C}_{6}\right) \) Nonnegativity: \[ \overline{X_{1} \geq 0, X_{2} \geq 0, X_{3} \geq 0,}, x_{4} \geq 0, X_{5} \geq 0, X_{6} \geq 0 \]