Home /
Expert Answers /
Statistics and Probability /
given-below-is-a-bivariate-distribution-for-the-random-variables-x-and-y-a-compute-th-pa843
(Solved): Given below is a bivariate distribution for the random variables \( x \) and \( y \). a. Compute th ...
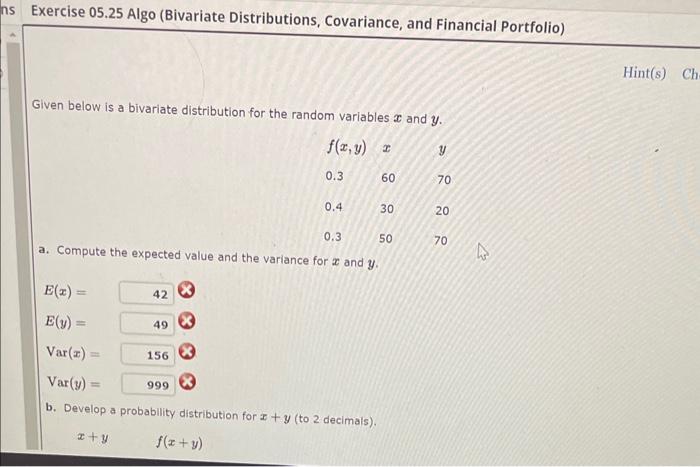
![b. Develop a probability distribution for \( x+y \) (to 2 decimals).
\[
x+y \quad f(x+y)
\]
Using the result of part (b), com](https://media.cheggcdn.com/study/58c/58ca344f-a1f3-4d5f-ac63-5201ce850475/image)
Given below is a bivariate distribution for the random variables \( x \) and \( y \). a. Compute the expected value and the variance for \( x \) and \( y \). \[ \begin{array}{l} E(x)= \\ E(y)= \\ \operatorname{Var}(x)= \\ \operatorname{Var}(y)= \end{array} \] b. Develop a probability distribution for \( x+y \) (to 2 decimals). \[ \begin{array}{ll} x+y & f(x+y) \end{array} \]
b. Develop a probability distribution for \( x+y \) (to 2 decimals). \[ x+y \quad f(x+y) \] Using the result of part (b), compute \( E(x+y) \) and \( \operatorname{Var}(x+y) \). c. Using the ret \( E(x+y)= \) \[ \operatorname{Var}(x+y)= \] d. Compute the covariance and correlation for \( x \) and \( y \). If required, round your answers to two decimal places. Covariance \( = \) Correlation \( = \) The random variables \( x \) and \( y \) are e. The variance of the sum of \( x \) and \( y \) is the sum of the individual variances. By how much? \( \operatorname{Var}(x+y) \) is greater than \( \operatorname{Var}(x)+\operatorname{Var}(y) \) by twice the covariance \( y \)
Expert Answer
Joint Probability: The joint distribution is a bivariate distribution of the random variables. The variance of the distribution must be a positive num