Home /
Expert Answers /
Calculus /
let-p-9-4-7-15-vec-y-1-t-2e-3t-4e-t-3e-3t-10e-t-vec-y-2-t-4e-3t-2-pa795
(Solved): Let P=[[9,-4,-7]] 15, vec(y)_(1)(t)=[[2e^(3t)+4e^(-t)]] 3e^(3t)+10e^(-t),vec(y)_(2)(t)=[[-4e^(3t)+2 ...
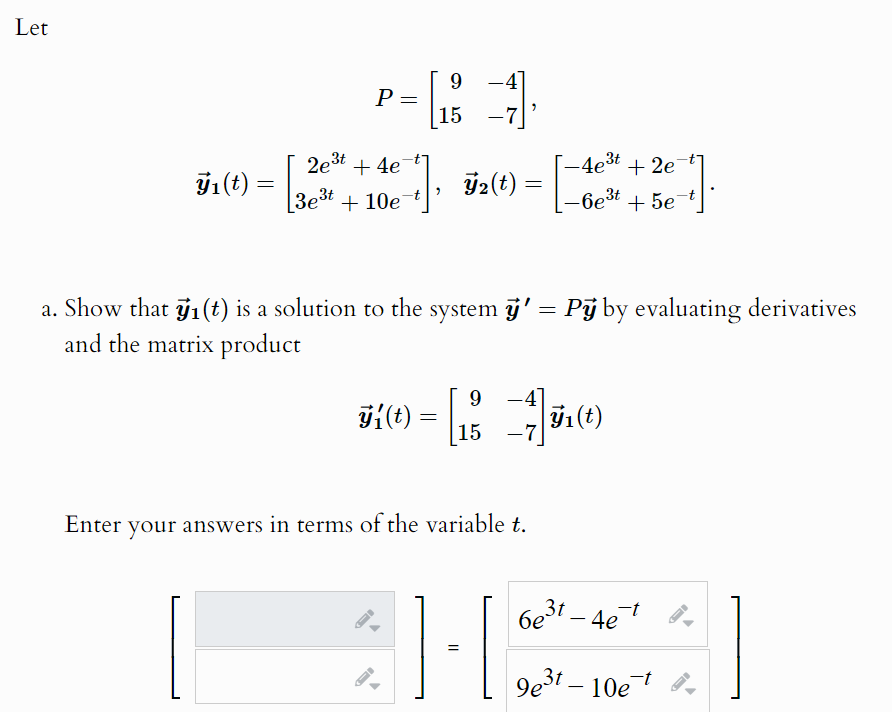
Let
P=[[9,-4,-7]]
15,
vec(y)_(1)(t)=[[2e^(3t)+4e^(-t)]]
3e^(3t)+10e^(-t),vec(y)_(2)(t)=[[-4e^(3t)+2e^(-t)]]
-6e^(3t)+5e^(-t).a. Show that
vec(y)_(1)(t)is a solution to the system
vec(y)^(')=Pvec(y)by evaluating derivatives and the matrix product
vec(y)_(1)^(')(t)=[[9,-4],[15,-7]]vec(y)_(1)(t)Enter your answers in terms of the variable
t.
[◻]=[[6e^(3t)-4e^(-t)],[9e^(3t)-10e^(-t)]]