(Solved): Macon Controls produces three different types of control units used to protect industrial equipment ...

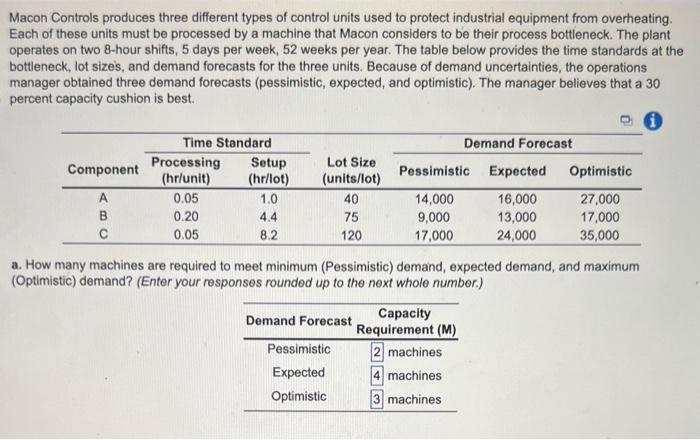
Macon Controls produces three different types of control units used to protect industrial equipment from overheating. Each of these units must be processed by a machine that Macon considers to be their process bottleneck. The plant operates on two 8-hour shifts, 5 days per week, 52 weeks per year. The table below provides the time standards at the bottleneck, lot sizes, and demand forecasts for the three units. Because of demand uncertainties, the operations manager obtained three demand forecasts (pessimistic, expected, and optimistic). The manager believes that a 30 percent capacity cushion is best. a. How many machines are required to meet minimum (Pessimistic) demand, expected demand, and maximum (Optimistic) demand? 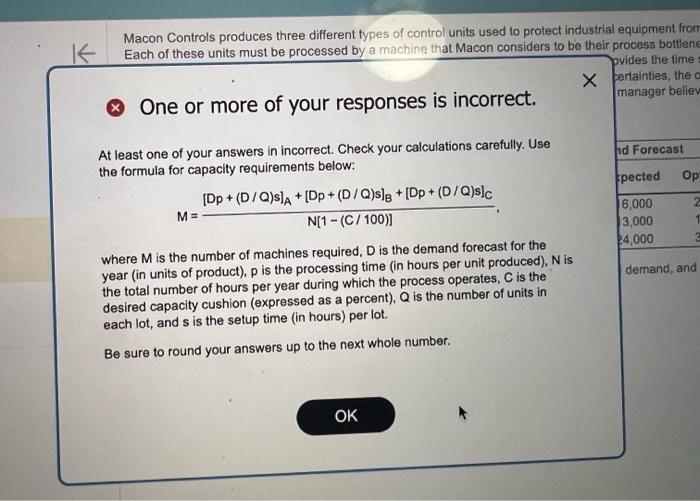(Enter your responses rounded up to the next whole number.) Macon Controls produces three different types of control units used to protect industriai equipment fron Each of these units must be processed by a machine that Macon considers to be their process bottlene * One or more of your responses is incorrect. At least one of your answers in incorrect. Check your calculations carefully. Use the formula for capacity requirements below: \[ M=\frac{[D p+(D / Q) s]_{A}+\left[D p+(D / Q) s_{B}+[D p+(D / Q) s]_{C}\right.}{N[1-(C / 100)]} . \] where \( M \) is the number of machines required, \( D \) is the demand forecast for the year (in units of product), \( p \) is the processing time (in hours per unit produced), \( N \) is the total number of hours per year during which the process operates, \( C \) is the demand, and desired capacity cushion (expressed as a percent), \( Q \) is the number of units in each lot, and \( s \) is the setup time (in hours) per lot. Be sure to round your answers up to the next whole number.