(Solved): Points A and D are connected to "ground" and is a distance L_(4) apart in the x-direction and L_(, ) ...
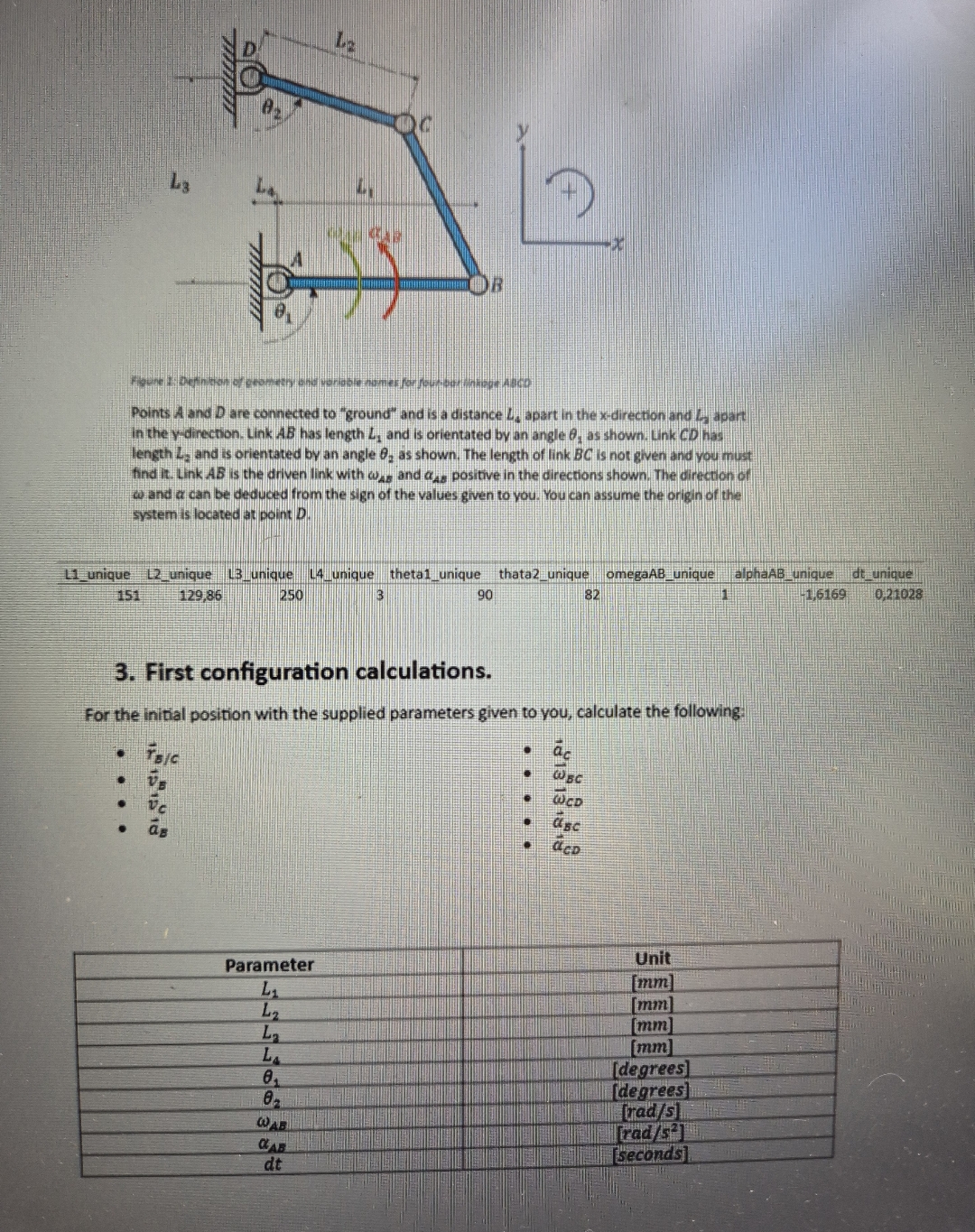
Points
Aand
Dare connected to "ground" and is a distance
L_(4)apart in the
x-direction and
L_(, )apart In the
y-direction. Link
ABhas length
L_(1)and is orientated by an angle
\theta _(1)as shown. Link
CDhas length
L_(2)and is orientated by an angle
\theta _(2)as shown. The length of link
BCis not given and you must find it. Link
ABis the driven link with
\omega _(As)and
\alpha _(As)positive in the directions shown. The direction of
\omega and
\alpha can be deduced from the sign of the values given to you. You can assume the origin of the system is located at point
D. \table[[11. unique,12 unique,13 _unique,L4_unique,thetal_unique,thata2 unique,omegaAB_unique,alphaAB,unique,dt_unique],[151,129,86,250,3,90,82,1,,1,6169,0,21028]] First configuration calculations. For the initial position with the supplied parameters given to you, calculate the following:
vec(r)_((E)/(C))
vec(v)_(B)
vec(v)_(c)
vec(a)_(B)
vec(a)_(c)
/bar (\omega )_(BC)
/bar (\omega )_(CD)
vec(a)_(BC)
vec(a)_(CD)