(Solved): Problem 1 In the flow-level system shown below The input is the flow rate Q(t)=Q+q(t), flow ...

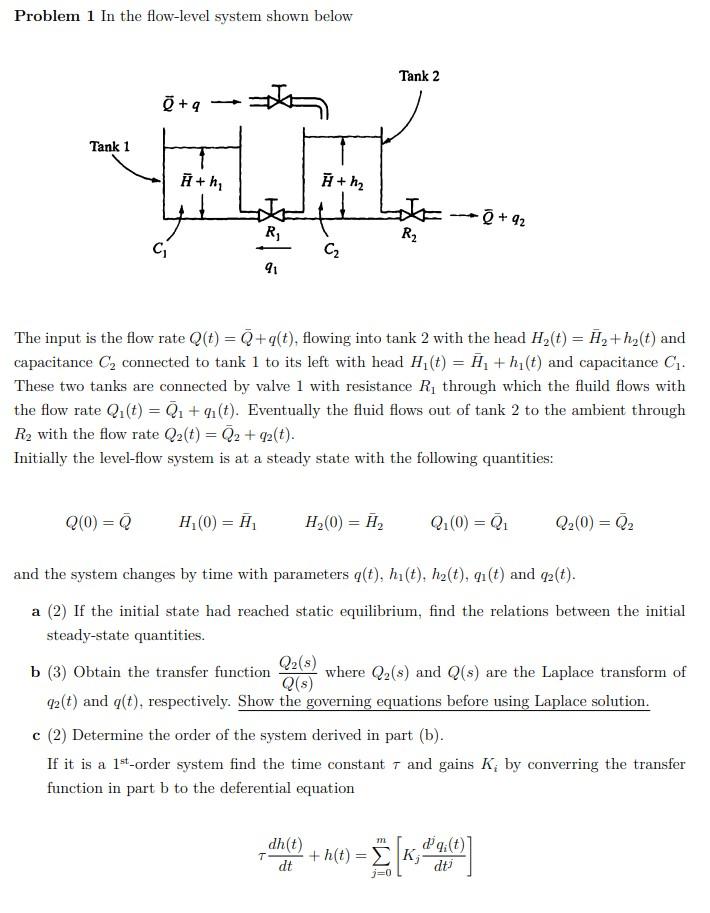
Problem 1 In the flow-level system shown below The input is the flow rate $$Q(t)=\stackrel{?}{Q}+q(t)$$, flowing into tank 2 with the head $$H_2(t)={\stackrel{?}{H}}_2+h_2(t)$$ and capacitance $$C_2$$ connected to tank 1 to its left with head $$H_1(t)={\stackrel{?}{H}}_1+h_1(t)$$ and capacitance $$C_1$$. These two tanks are connected by valve 1 with resistance $$R_1$$ through which the fluild flows with the flow rate $$Q_1(t)={\stackrel{?}{Q}}_1+q_1(t)$$. Eventually the fluid flows out of tank 2 to the ambient through $$R_2$$ with the flow rate $$Q_2(t)={\stackrel{?}{Q}}_2+q_2(t)$$. Initially the level-flow system is at a steady state with the following quantities: $$Q(0)=\stackrel{?}{Q}{H}_1(0)={\stackrel{?}{H}}_1{H}_2(0)={\stackrel{?}{H}}_2{Q}_1(0)={\stackrel{?}{Q}}_1{Q}_2(0)={\stackrel{?}{Q}}_2$$ and the system changes by time with parameters $$q(t),h_1(t),h_2(t),q_1(t)$$ and $$q_2(t)$$. a (2) If the initial state had reached static equilibrium, find the relations between the initial steady-state quantities. b (3) Obtain the transfer function $$\frac{Q_2(s)}{Q(s)}$$ where $$Q_2(s)$$ and $$Q(s)$$ are the Laplace transform of $$q_2(t)$$ and $$q(t)$$, respectively. Show the governing equations before using Laplace solution. c (2) Determine the order of the system derived in part (b). If it is a $$1^{\text{st }}$$-order system find the time constant $$?$$ and gains $$K_i$$ by converring the transfer function in part $$b$$ to the deferential equation $$?\frac{dh(t)}{dt}+h(t)=\underset{j=0}{\overset{m}{?}}\left[K_j\frac{d^j{q}_i(t)}{d{t}^j}\right]$$ and if is $$2^{\text{nd }}$$-order system find the (undamped) natural frequency $${?}_n$$, damping ratio $$?$$ and gains $$K_i$$ by converring the transfer function in part b to the deferential equation $$\frac{d^{2}h(t)}{d{t}^2}+2?{?}_n\frac{dh(t)}{dt}+{?}_n^{2}h(t)=\underset{j=0}{\overset{m}{?}}\left[{?}_n^2{K}_j\frac{d^j{q}_i(t)}{d{t}^j}\right]$$ d (1) If the change in inflow rate $$q(t)$$ is a step function, $$q(t)=\stackrel{?}{q}1(t)$$, where the constant $$\stackrel{?}{q}$$ is the step change in inflow rate, find the steady-state value $$q_{2,ss}=\underset{t??}{\lim }{q}_2(t)$$