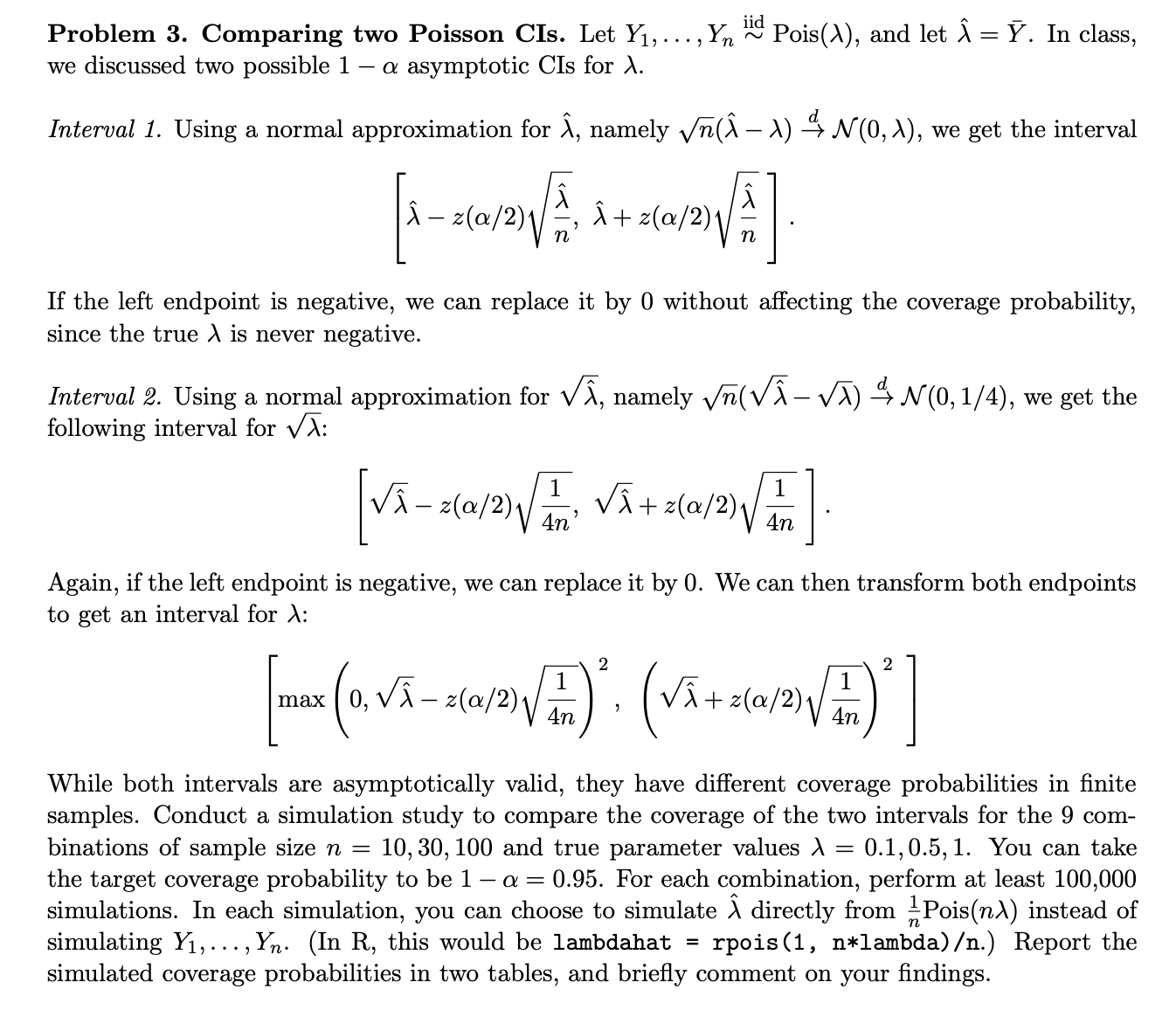
(Solved): Problem 3. Comparing two Poisson CIs. Let Y_(1),dots,Y_(n)^( iid )Pois(\lambda ), and let hat(\l ...
Problem 3. Comparing two Poisson CIs. Let
Y_(1),dots,Y_(n)∼^( iid )Pois(\lambda ), and let
hat(\lambda )()/(b)=ar (Y). In class, we discussed two possible
1-\alpha asymptotic CIs for
\lambda . Interval 1. Using a normal approximation for
hat(\lambda ), namely
\sqrt(n)(hat(\lambda )-\lambda )->dN(0,\lambda ), we get the interval
[(hat(\lambda ))-z((\alpha )/(2))\sqrt(((hat(\lambda )))/(n)),(hat(\lambda ))+z((\alpha )/(2))\sqrt(((hat(\lambda )))/(n))].If the left endpoint is negative, we can replace it by 0 without affecting the coverage probability, since the true
\lambda is never negative. Interval 2. Using a normal approximation for
\sqrt(h)at(\lambda ), namely
\sqrt(n)(\sqrt(h)at(\lambda )-\sqrt(\lambda ))->dN(0,(1)/(4)), we get the following interval for
\sqrt(\lambda ):
[\sqrt(h)at(\lambda )-z((\alpha )/(2))\sqrt((1)/(4n)),\sqrt(h)at(\lambda )+z((\alpha )/(2))\sqrt((1)/(4n))].Again, if the left endpoint is negative, we can replace it by 0 . We can then transform both endpoints to get an interval for
\lambda :
max(0,\sqrt(h)at(\lambda )-z((\alpha )/(2))\sqrt((1)/(4n)))^(2),(\sqrt(h)at(\lambda )+z((\alpha )/(2))\sqrt((1)/(4n)))^(2)While both intervals are asymptotically valid, they have different coverage probabilities in finite samples. Conduct a simulation study to compare the coverage of the two intervals for the 9 com- binations of sample size
n=10,30,100and true parameter values
\lambda =0.1,0.5,1. You can take the target coverage probability to be
1-\alpha =0.95. For each combination, perform at least 100,000 simulations. In each simulation, you can choose to simulate
hat(\lambda )directly from
(1)/(n)Pois(n\lambda )instead of simulating
Y_(1),dots,Y_(n). (In R, this would be lambdahat
=rpois(1,
n*lambda)/n.) Report the simulated coverage probabilities in two tables, and briefly comment on your findings.