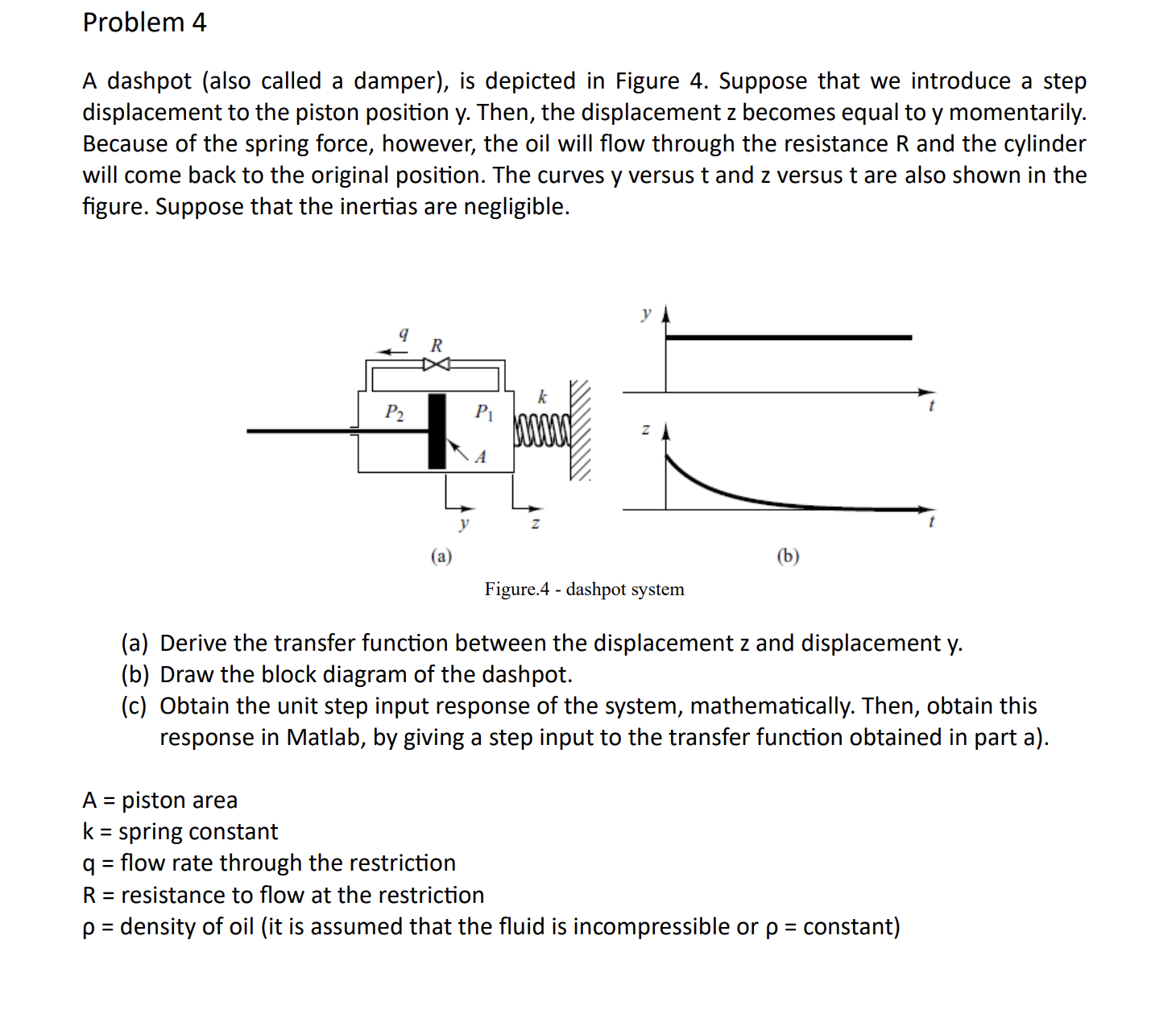
(Solved): Problem 4 A dashpot (also called a damper), is depicted in Figure 4. Suppose that we introduce a ste ...

Problem 4 A dashpot (also called a damper), is depicted in Figure 4. Suppose that we introduce a step displacement to the piston position y . Then, the displacement z becomes equal to y momentarily. Because of the spring force, however, the oil will flow through the resistance \( R \) and the cylinder will come back to the original position. The curves \( y \) versus \( t \) and \( z \) versus \( t \) are also shown in the figure. Suppose that the inertias are negligible. (a) Derive the transfer function between the displacement \( z \) and displacement \( y \). (b) Draw the block diagram of the dashpot. (c) Obtain the unit step input response of the system, mathematically. Then, obtain this response in Matlab, by giving a step input to the transfer function obtained in part a). A = piston area \( \mathrm{k}= \) spring constant \( q= \) flow rate through the restriction \( R= \) resistance to flow at the restriction \( \rho= \) density of oil (it is assumed that the fluid is incompressible or \( \rho= \) constant)