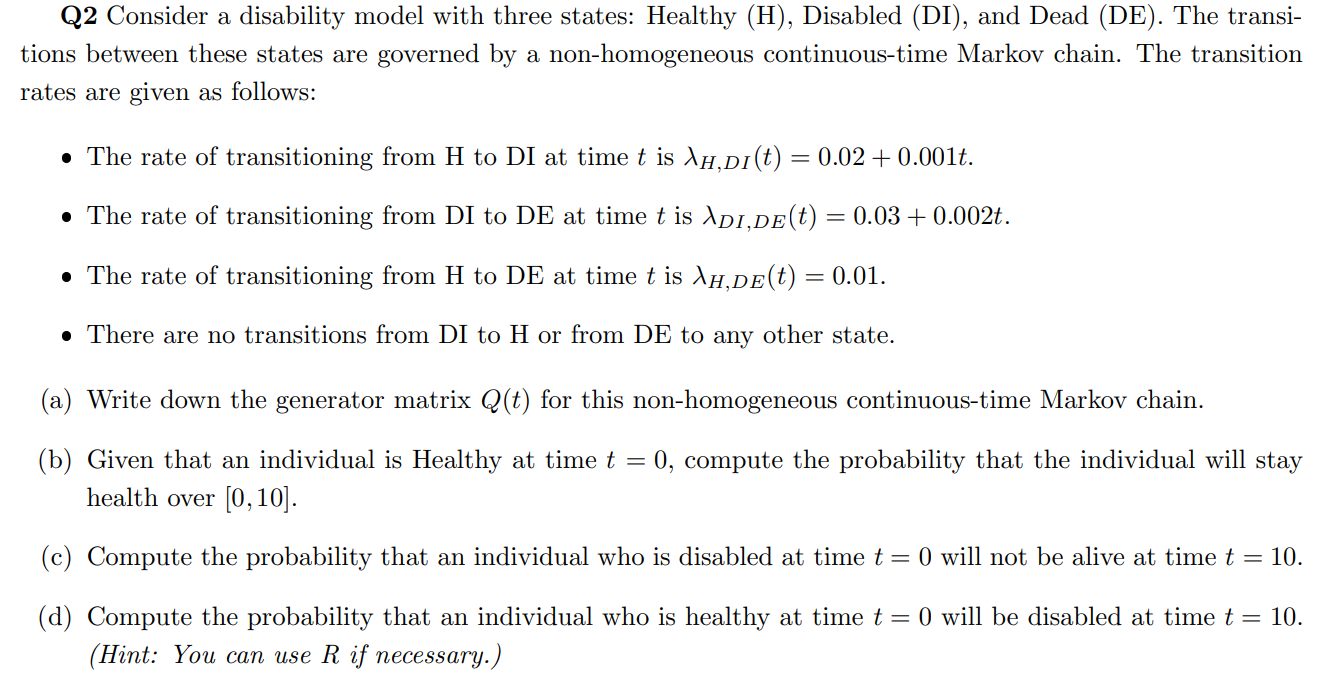
(Solved): Q2 Consider a disability model with three states: Healthy (H), Disabled (DI), and Dead (DE). The tr ...
Q2 Consider a disability model with three states: Healthy
(H), Disabled (DI), and Dead (DE). The transi- tions between these states are governed by a non-homogeneous continuous-time Markov chain. The transition rates are given as follows: The rate of transitioning from H to DI at time
tis
\lambda _(H,DI)(t)=0.02+0.001t. The rate of transitioning from DI to DE at time
tis
\lambda _(DI,DE)(t)=0.03+0.002t. The rate of transitioning from H to DE at time
tis
\lambda _(H,DE)(t)=0.01. There are no transitions from DI to H or from DE to any other state. (a) Write down the generator matrix
Q(t)for this non-homogeneous continuous-time Markov chain. (b) Given that an individual is Healthy at time
t=0, compute the probability that the individual will stay health over
0,10. (c) Compute the probability that an individual who is disabled at time
t=0will not be alive at time
t=10. (d) Compute the probability that an individual who is healthy at time
t=0will be disabled at time
t=10. (Hint: You can use
Rif necessary.)