(Solved): , Sound waves undergo reflection and refraction, much as electromagnetic waves do. Here is one prac ...

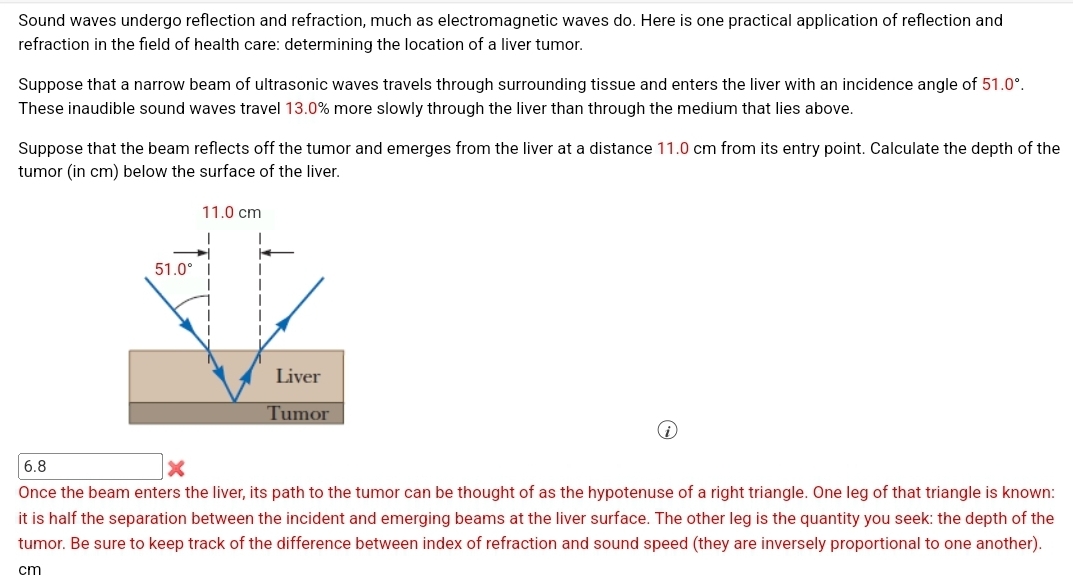
, Sound waves undergo reflection and refraction, much as electromagnetic waves do. Here is one practical application of reflection and refraction in the field of health care: determining the location of a liver tumor. Suppose that a narrow beam of ultrasonic waves travels through surrounding tissue and enters the liver with an incidence angle of

. These inaudible sound waves travel

more slowly through the liver than through the medium that lies above. Suppose that the beam reflects off the tumor and emerges from the liver at a distance 11.0 cm from its entry point. Calculate the depth of the tumor (in cm ) below the surface of the liver. (i) Once the beam enters the liver, its path to the tumor can be thought of as the hypotenuse of a right triangle. One leg of that triangle is known: it is half the separation between the incident and emerging beams at the liver surface. The other leg is the quantity you seek: the depth of the tumor. Be sure to keep track of the difference between index of refraction and sound speed (they are inversely proportional to one another). cm