Suppose C is the curve r(t)=(:2t,2t^(3):), for 0

Question

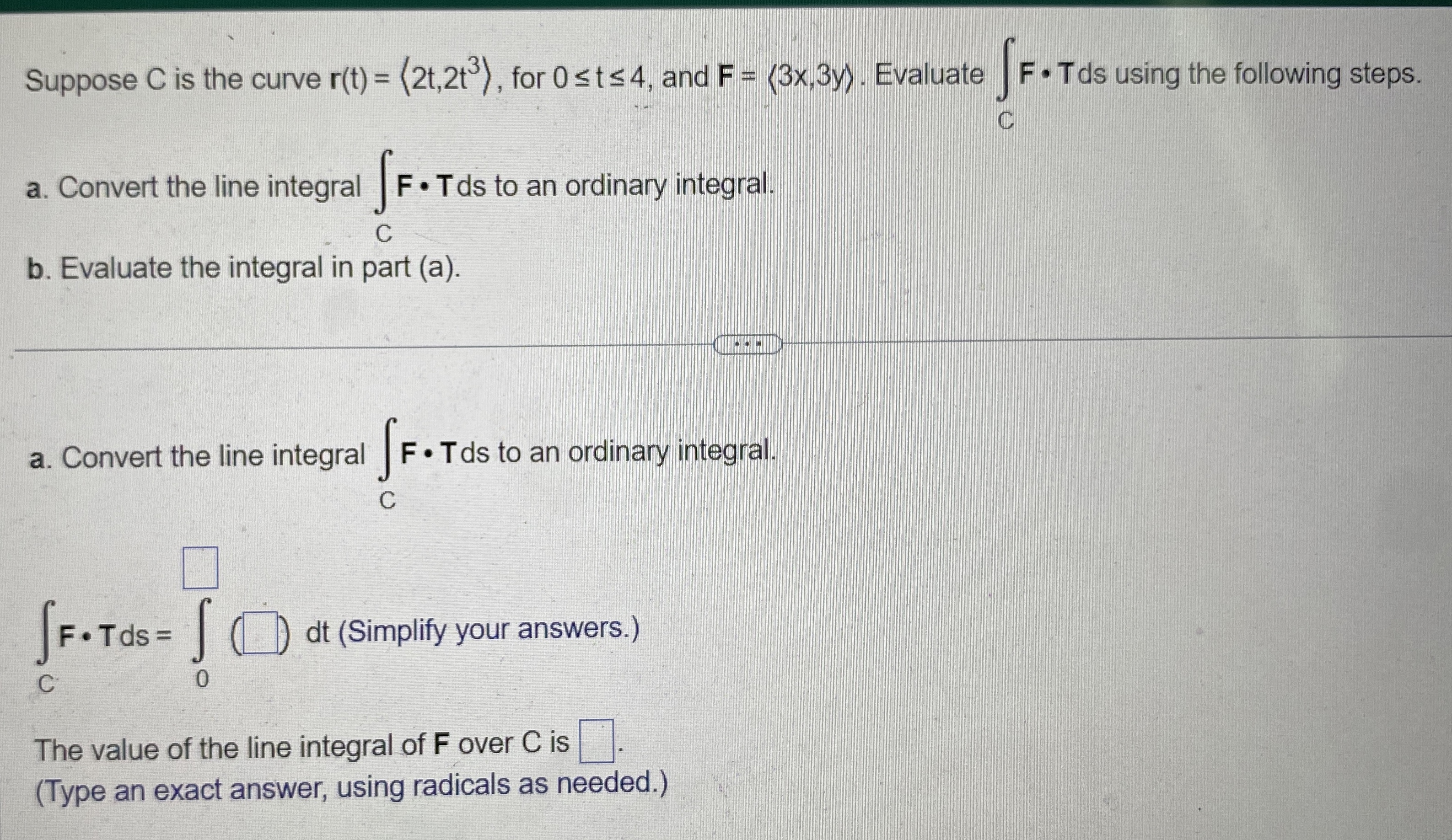
Suppose C is the curve r(t)=(:2t,2t^(3):), for 0<=t<=4, and F=(:3x,3y:). Evaluate  int_C F*Tds using the following steps. a. Convert the line integral  int_C F*Tds to an ordinary integral. b. Evaluate the integral in part (a). a. Convert the line integral  int_C F*Tds to an ordinary integral.  int_C F*Tds= int_0 dt (Simplify your answers.)  The value of the line integral of F over C is  (Type an exact answer, using radicals as needed.)

Accepted Answer

Expert Answer to - Suppose C is the curve r(t)=(:2t,2t^(3):), for 0<=t<=4, and F=(:3x,3y:). Evaluate  int_C F*Tds

Answer

Solution for - Suppose C is the curve r(t)=(:2t,2t^(3):), for 0<=t<=4, and F=(:3x,3y:). Evaluate  int_C F*Tds

Answer

This an additional answer to - Suppose C is the curve r(t)=(:2t,2t^(3):), for 0<=t<=4, and F=(:3x,3y:). Evaluate  int_C F*Tds

(Solved): Suppose C is the curve r(t)=(:2t,2t^(3):), for 0<=t<=4, and F=(:3x,3y:). Evaluate \int_C F*Tds ...

View Expert Answer

Expert Answer

Buy This Answer $5

Place Order

We Provide Services Across The Globe