Home /
Expert Answers /
Calculus /
we-are-asked-to-find-the-maclaurin-series-for-a-function-involving-cos-x-recall-the-maclaurin-ser-pa984
(Solved): We are asked to find the Maclaurin series for a function involving cos(x). Recall the Maclaurin ser ...
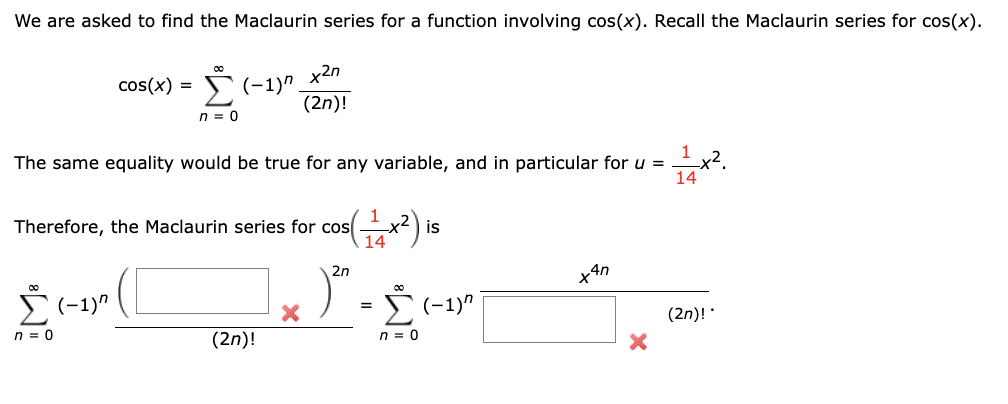
We are asked to find the Maclaurin series for a function involving
cos(x). Recall the Maclaurin series for
cos(x).
cos(x)=\sum_(n=0)^(\infty ) (-1)^(n)(x^(2n))/((2n)!)The same equality would be true for any variable, and in particular for
u=(1)/(14)x^(2). Therefore, the Maclaurin series for
cos((1)/(14)x^(2))is
\sum_(n=0)^(\infty ) (-1)^(n)((x)^(2n))/((2n)!)=\sum_(n=0)^(\infty ) (-1)^(n)(x^(4n))/((2n)!)