(Solved): - where not met in 200 iterations Recall the integration by parts formuala: \[ \text { int } f(x) ...

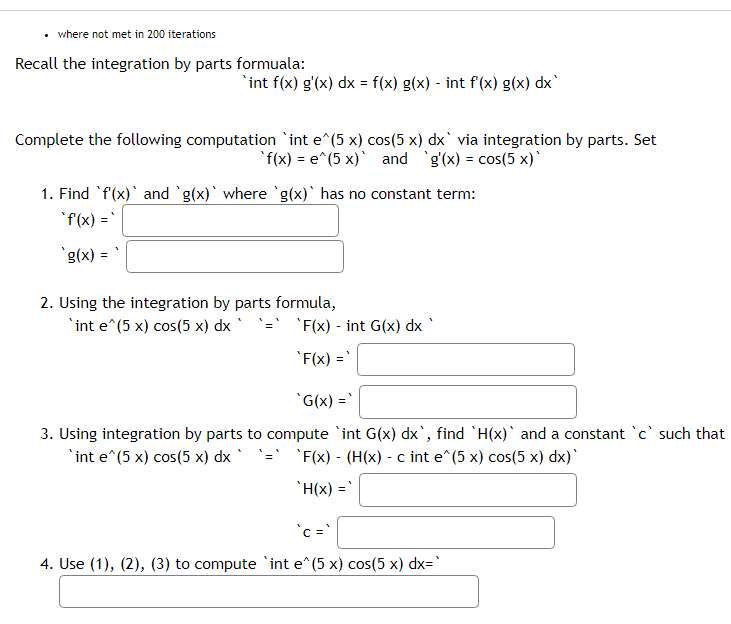
- where not met in 200 iterations Recall the integration by parts formuala: \[ \text { int } f(x) g^{\prime}(x) d x=f(x) g(x) \text { - int } f^{\prime}(x) g(x) d x \text { ' } \] Complete the following computation 'int \( \mathrm{e}^{\wedge}(5 \mathrm{x}) \cos (5 \mathrm{x}) \mathrm{dx} \) ' via integration by parts. Set \[ ' f(x)=e^{\wedge}(5 x)^{\prime} \text { and } \quad{ }^{\prime} g^{\prime}(x)=\cos (5 x)^{\prime} \] 1. Find ' \( f^{\prime}(x)^{\prime} \) and ' \( g(x)^{\prime} \) where ' \( g(x)^{\prime} \) has no constant term: \[ \begin{array}{l} ' f^{\prime}(x)=' \\ ' g(x)=' \end{array} \] 2. Using the integration by parts formula, \[ \begin{array}{c} \text { int } \mathrm{e}^{\wedge}(5 x) \cos (5 x) d x{ }^{\prime}={ }^{\prime} \quad F(x)-\text { int } G(x) d x ' \\ ' F(x)=' \\ ' G(x)=' \end{array} \] 3. Using integration by parts to compute 'int \( G(x) d x^{\prime} \), find ' \( H(x)^{\prime} \) and a constant ' \( c \) ' such that 'int \( \mathrm{e}^{\wedge}(5 \mathrm{x}) \cos (5 \mathrm{x}) \mathrm{dx} \) ' \( ={ }^{\prime} \quad ` \mathrm{~F}(\mathrm{x})-\left(\mathrm{H}(\mathrm{x})-\mathrm{c} \text { int } \mathrm{e}^{\wedge}(5 \mathrm{x}) \cos (5 \mathrm{x}) \mathrm{dx}\right)^{\prime} \) \[ \text { ' } \mathrm{H}(\mathrm{x})=\text { ' } \] \[ \text { ' } \mathrm{C}=\text { ' } \] 4. Use (1), (2), (3) to compute 'int \( \mathrm{e}^{\wedge}(5 \mathrm{x}) \cos (5 \mathrm{x}) \mathrm{dx}= \) '