Home /
Expert Answers /
Calculus /
xy-39-1-x-y-e-x-sin2x-ydx-4-x-y-6-dy-0-ydx-ye-y-2x-dy-cosx-dy-dx-sinx-y-1-cos-2-xsinx-pa677
(Solved): xy^(')+(1+x)y=e^(-x)sin2x ydx-4(x+y^(6))dy=0 ydx=(ye^(y)-2x)dy cosx(dy)/(dx)+(sinx)y=1 cos^(2)xsinx( ...
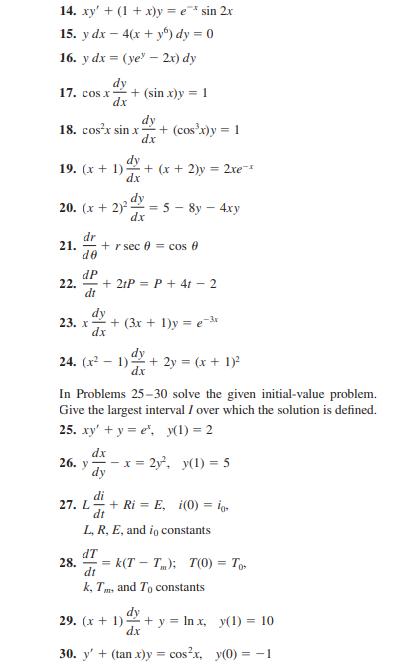
xy^(')+(1+x)y=e^(-x)sin2x
ydx-4(x+y^(6))dy=0
ydx=(ye^(y)-2x)dy
cosx(dy)/(dx)+(sinx)y=1
cos^(2)xsinx(dy)/(dx)+(cos^(3)x)y=1
(x+1)(dy)/(dx)+(x+2)y=2xe^(-x)
(x+2)^(2)(dy)/(dx)=5-8y-4xy
(dr)/(d\theta )+rsec\theta =cos\theta
(dP)/(dt)+2tP=P+4t-2
x(dy)/(dx)+(3x+1)y=e^(-3x)
(x^(2)-1)(dy)/(dx)+2y=(x+1)^(2)
In Problems 25-30 solve the given initial-value problem.
Give the largest interval I over which the solution is defined.
xy^(')+y=e^(x),y(1)=2
y(dx)/(dy)-x=2y^(2),y(1)=5
L(di)/(dt)+Ri=E,i(0)=i_(0),
L,R,E, and i_(0) constants
(dT)/(dt)=k(T-T_(m));,T(0)=T_(0),
k,T_(m), and T_(0) constants
(x+1)(dy)/(dx)+y=lnx,y(1)=10
y^(')+(tanx)y=cos^(2)x,y(0)=-1